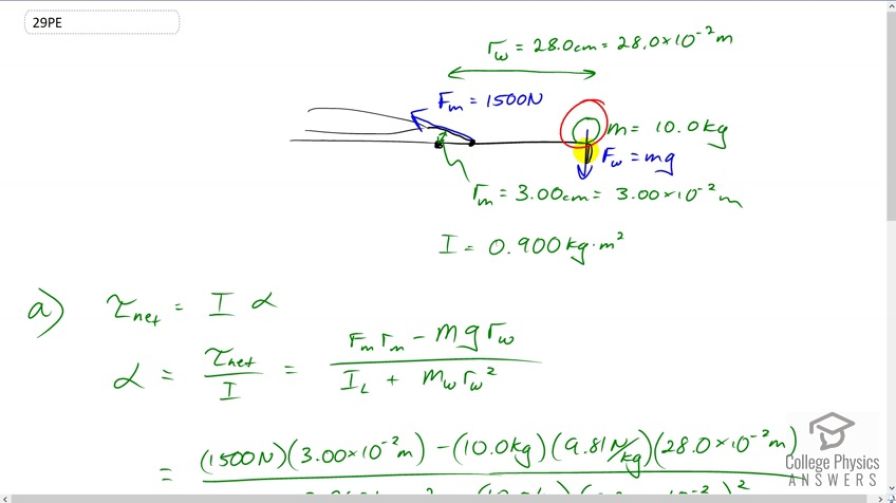
a)
b)
Note: This video assumes there isn't any torque due to the weight of the lower leg. With the weight of the leg included in the torque calculation, the answer to part (a) would be . For all the questions in chapter 10, the torque due to the weight of a limb is ignored. Like ignoring friction, this is a simplification done to make real-world complexity more manageable. For instance, for part (b) of this question, it would be challenging to account for how the torque due to gravity on the lower leg would change as the angle of the lower leg changes. As the lower leg is lifted, the perpendicular component of the lever arm of gravity changes. Dealing with quantities that change gradually like this is the subject of calculus and is beyond the scope of this textbook.Solution video
OpenStax College Physics, Chapter 10, Problem 29 (Problems & Exercises)
Calculator Screenshots
Comments
In calculating the net torque why wasn’t the weight of the bottom part of the leg to its center of mass considered?
Hi djab, this is a great question. I think you're right to consider the weight of the leg, and perhaps this video deserves a re-do to take the torque due to the weight of the leg into account. Assuming the lower leg has mass uniformly distributed and is like a rod rotating about an axis through one end (the knee in this case), then its moment of inertia would be , giving a mass for the lower leg of 34.44 kg. With the weight assumed to be acting at the center of the lower leg, at 1:42 you could introduce a term for the clockwise torque due to the weight of the leg which would subtract from the counterclockwise torque due to the muscle. The new answer for the angular acceleration in part (a) would be 7.55 rad/s, which would also change the answer for the work done in part (b). I'll put a note in the final answer to refer to this comment, and I've flagged this video for a re-do.
All the best,
Shaun
This video is not going to get a re-do since it makes a reasonable simplification that's common in this chapter.


