Question
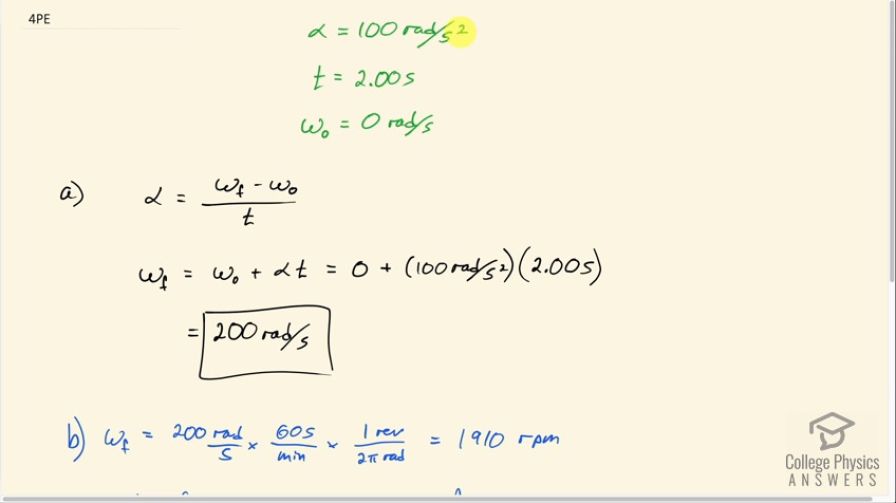
You are told that a basketball player spins the ball with an angular acceleration of . (a) What is the ball’s final angular velocity if the ball starts from rest and the acceleration lasts 2.00 s? (b) What is unreasonable about the result? (c) Which premises are unreasonable or inconsistent?
Final Answer
- . This speed is unreasonably high. The friction against the player's finger would burn their finger, not to mention the difficulty of accelerating a ball to this speed in the first place.
- The angular acceleration can't be so large.
Solution video
OpenStax College Physics, Chapter 10, Problem 4 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
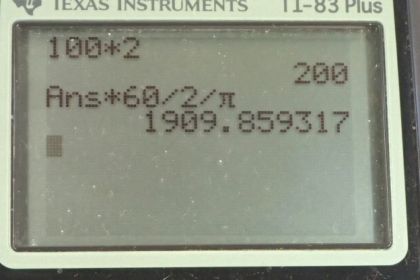
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A basketball player supposedly spins a basketball with an angular acceleration of 100 radians per second per second and does this for 2.00 seconds. And starting from an initial angular velocity of zero, what will the final angular velocity be? Well, angular acceleration is the change in angular velocity divided by time and we can multiply both sides by t and then add ω naught to both sides and switch the sides around and we get that the final angular velocity is the initial angular velocity plus angular acceleration multiplied by time. So a 0 plus 100 radians per second squared times 2.00 seconds which is 200 radians per second. To give some perspective on what that means, let's convert it into rpm or revolutions per minute so multiply it by 60 seconds per minute and then multiply it by 1 revolution for every 2π radians and we are left with revolutions per minute which is 1910. That is a very large number and the friction of a ball spinning that fast would burn the players' finger never mind how they would be capable of spinning it so fast in the first place. So this angular acceleration can't be so large.