Question
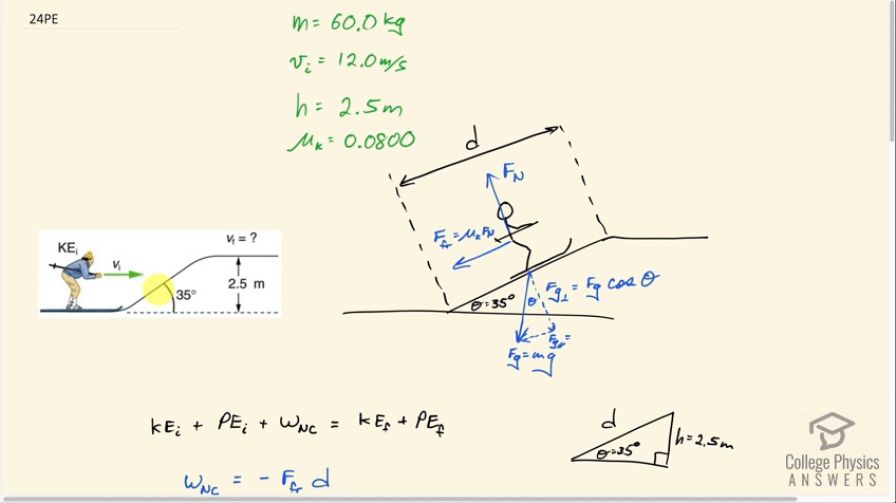
A 60.0-kg skier with an initial speed of 12.0 m/s coasts up a 2.50-m-high rise as shown in Figure 7.40. Find her final speed at the top, given that the coefficient of friction between her skis and the snow is 0.0800. (Hint: Find the distance traveled up the incline assuming a straight-line path as shown in the figure.)
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 7, Problem 24 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This skier has some initial velocity of 12.0 meters per second and then they encounter a 35 degree slope. They go up the slope—a total height of 2.5 meters—and then they are skiing horizontally at the top and the question is what speed will they have on this top level assuming that there's a certain amount of friction due to a coefficient of friction of 0.0800 along this slope here. So we are going to figure out how much work is done by the non-conservative force, namely friction, along this slope and then that work is going to be the energy that is taken away from kinetic and potential energy that the skier had initially and then when they are at the top here, they will be left with just some potential and kinetic energy but the total will not be the total initial kinetic and potential energy because some of it will be taken away by the work done by the non-conservative force. There's a plus sign here which suggests that maybe this thing is adding energy but in fact, it's going to be negative because the friction and the displacement are in opposite directions and so this work will be negative. Okay! So the distance that they travel along the slope we can figure that out from this triangle here: we know that sin of this angle Θ is the opposite divided by the hypotenuse so that's h over d and then we can solve for d by multiplying both sides by d over sin Θ. We cancel the sin Θ's there and cancel the d's over here and we have d equals h over sin Θ. Okay! This is important because we are gonna substitute in for d here in this formula for the work done by the non-conservative force which is going to be the negative and I put negative there just because, you know, work is force times displacement times cosine of the angle between them and cos of 180 degrees is negative 1 or you could think of it as the displacement and the force are in opposite directions and that's why there's a negative there. Alright. The other factor here is this friction force and it's going to be their coefficient of friction multiplied by the normal force applied by the slope and the normal force is going to equal the component of gravity that is perpendicular to the slope. So gravity is straight down but there's this component here which is the force of gravity times cos of Θ which will be the perpendicular component of gravity and these two have to be equal because the skier is not accelerating perpendicular to the slope. Okay! So we have μ kF N and then substituting in the perpendicular component of gravity in place of normal force and we have μ k is times mgcos Θ is the friction force. So we can substitute for both friction force— μ kmgcos Θ—and substitute for the distance along the slope— which is h over sin Θ— and then we have this formula for the work done by friction. It's gonna be negative times coefficient of friction times mgh divided by tangent Θ— because cos over sin... there's an identity which says that cos Θ divided by sin Θ is the reciprocal of tan Θ. Okay! So all of this gets plugged into the work done by the non-conservative force here in our conservation of energy formula and then we can start plugging in for the other terms as well. So the initial kinetic energy is one-half mass times initial velocity squared plus the initial potential energy and we'll say that that is zero because we'll define this to be our reference level where h equals 0 so mgh would be mg times 0 and then adding to that... that should be a minus or maybe a plus for the formula and then a minus for the substitution but let's just resolve it into a single operation which is minus. Okay! And that equals one-half mv f squared plus mgh— this is the potential energy at the top of the slope and the kinetic energy at the top of the slope and this v f is what we are ultimately trying to find. So we'll make things look a little bit simpler by multiplying everything by 2 over m; it's kind of messy having fractions so we'll get rid of the fractions by multiplying by 2 and then we'll also get rid of the m which is a factor in all of the terms. So we are left with, after you switch the sides around, v f squared plus 2gh—the m is canceled and this 2 is canceled but the 2 appears here because it has to get distributed among both terms into the brackets— and that equals v initial squared minus 2μ kgh over tan Θ. I made a mistake here with the plus sign but nevertheless I corrected it anyway and it's correctly written as a minus here. Okay! So we are gonna subtract 2gh from both sides to solve for v f squared and we have this line and I factored out this common factor 2gh from both of these terms and it becomes minus 2gh times 1 plus μ k over tan Θ and then take the square root of both sides and that's all the algebra that we need to do. So v f is the square root of v initial squared minus 2gh times 1 plus μ k over tan Θ. So that's the square root of 12.0 meters per second squared minus 2 times 9.80 meters per second squared times 2.5 meters up the slope—that's the height of the slope— times 1 plus 0.0800—coefficient of friction— divided by tan of the slope angle of 35 degrees and this gives 9.5 meters per second. Now we are expecting some answer that is less than the initial speed so if we had had an answer more than 12, we would have said that doesn't pass the reality test but something less than 12 does. So 9.5 is plausibly the correct answer.

