OpenStax College Physics for AP® Courses, Chapter 7, Problem 41 (Problems & Exercises)

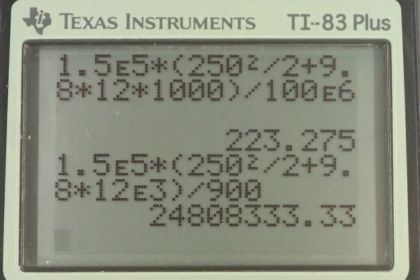
This is College Physics Answers with Shaun Dychko. We have a plane with a mass of 1.5 times ten to the five kilograms that is going to be taking off, starting at rest and reaching a final speed of 250 meters per second. It'll reach a height of 12 kilometers above the ground. The engines powering the plane have a power of 100 megawatts and the prefix mega mean times ten to the six, so we re-write this as 100 times ten to the six watts. Our job is to figure out how long will it take the plane to reach this height going at that speed given these engines and that mass. So power is the work or energy required to do whatever is being done, lifting up the plane in this case, dividing by the time it takes to do it. So, we'll multiply both sides by t over p to solve for time. So time is the work done divided by the power and we know that the initial total energy, total kinetic energy and potential energy initially, both of which are zero by the way because the plane is not moving and it starts on the ground at zero height. Then plus this work done by the motors, all of that energy has to equal the total final mechanical energy, kinetic and potential. So, we can see from this conservation of energy formula that the work done by the motors is going to have to equal the total final mechanical energy because this energy has to come from somewhere and it's coming from the motors. So, we'll replace the work done by the motors with kinetic energy final plus potential energy final here, then substitute for those in turn. So, final kinetic energy is one half mass of the plane times its final speed squared, plus its final potential energy being the mass of the plane times gravitational field strength times the height. We can factor out the m just to be a bit more concise in the way we write the formula. So we have m times bracket v f squared over two plus gh all over p. The substituting numbers, 1.5 times ten to the five kilograms times 250 meters per second squared, divided by two, plus 9.8 times 12 kilometers converted into meters, so times 1,000 meters per kilometer, divided by 100 times ten to the six watts which gives 223 seconds is the theoretical -- it's basically the minimum time that would be theoretically possible for the plane to reach that height with that speed. Now friction is going to be in play of course in the real world so the time will actually take longer. But we're not going to consider friction until part C. So, in part B we're still in an ideal case with no friction but we're told that the time is in fact 900 seconds. It is not the 223 seconds that we calculated. So given that it's 900 seconds, what must the power output of the motors be? So, we have our same formula from up here -- well, we've rearranged this -- this equals t by the way, we rearrange this to solve for P. So we can multiply this by P over t and this by P over t and the t's would cancel and we're left with P there. The P's would cancel over here being left with t on the bottom there. That will give us this formula here which is another way of saying the power is the total final mechanical energy divided by time. So we have the mass times the speed squared over two plus 9.8 times the height, all divided by 900 seconds, giving us 24.8 megawatts must be the power of the motors. Since it took longer that 223 we're now calculating a power output of the motors that is less than what we had assumed in part A. In part A we had assumed 100 megawatts but now in part B we're finding that given the time is longer than 223, the power is less than 100. Then in part C, we're told that given this power that we calculated in part B and given that the time the plane takes is 1200 seconds, so in part B we have t equals 1200 seconds, what must have the work done by friction have been and -- well, I mean that's part of what we calculate but the more important thing is what is the force due to friction? So, it's a bit of work to figure that out because well, we know that the total work done by the motor has to account for the kinetic energy that the plane has plus the potential energy that the plane has, plus all of the energy that's going to be lost due to friction which is the force of friction multiplied by the distance over which the plane has traveled. All of those three energies have to be -- you know, they have to come from somewhere and they come from, well, they come from chemical energy of the gasoline or the jet fuel in the motors. But in other words, these three energies are in total provided by the work done by the motor. Then, we're going to solve this for the force of friction eventually. So first we'll multiply both sides by t here and we have power times time equals kinetic plus potential plus the work done by friction. Then we'll get this term isolated by itself by subtracting kinetic and potential from both sides and then switching the sides around. We have force of friction times distance equals power times time minus the final kinetic and minus final potential energies, and then divide both sides by d and you're left with this line here. So there is more work to do yet though because we don't know what this dis so we have to calculate it based on our kinematics formulas. This one works nicely. We have that the average velocity, the final velocity plus initial velocity divided by two, multiplied by time is going to equal the distance. Since the initial speed is zero we can simplify this to be v f t over two and so we'll substitute that in for d in this line here. Now, I don't like dividing fractions by fractions and so instead I'm going to multiply by the reciprocal. So instead of dividing by d I'm multiplying by the reciprocal of d so I'm multiplying by two over v f t and then we end up with this line here. Now we know everything in that equation and we plug in numbers and get our answer. So we have two times 24.808 times ten to the six watts, this is the power calculated in part B with extra digits in order to avoid intermediate rounding error, multiplied by 1200 seconds, minus 1.5 times the mass of 1.5 times ten to the five kilograms, times 250 meters per second squared, minus the mass times 9.8 times 12 kilometers, and all divided by 250 meters per second times 1200 seconds. This means the force due to friction is 4.96 times ten to the four newtons.