OpenStax College Physics for AP® Courses, Chapter 7, Problem 34 (Test Prep for AP® Courses)

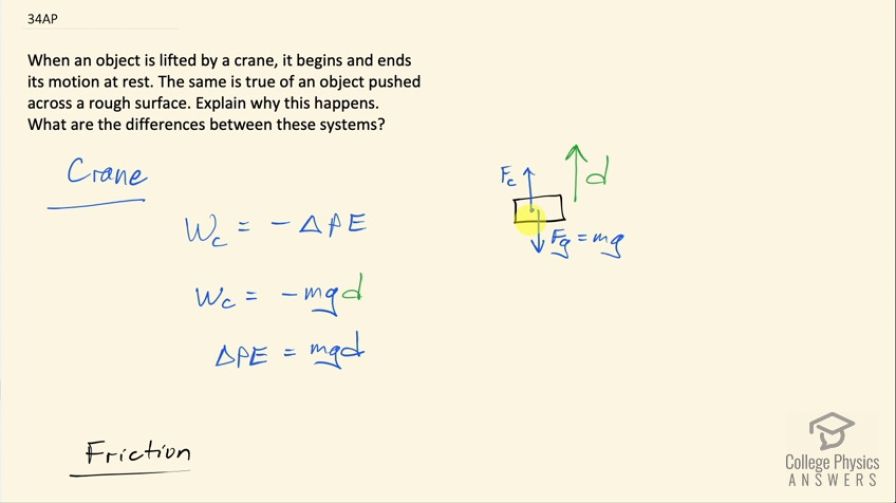
This is College Physics Answers with Shaun Dychko. A crane is lifting this object and the object begins at rest we are told and so for a very short, negligible period of time, this crane force upwards has to exceed gravity downwards in order to begin moving this object up in order to accelerate it up in other words but then shortly after, it returns to a magnitude equal to gravity so that it goes up at constant speed and then there must be some time when this crane force is reduced so that the thing slows down but then comes to a rest only if the crane force returns to the same magnitude as the gravity. So that's... a walkthrough of what's happening here as this object goes up and another thing is happening is that the object is gaining gravitational potential energy and we know that because this force downwards due to gravity is a conservative force which is to say that the work done by this force depends only on the position of the object not on whether it goes in a curved path or whether it goes in a straight path either way, gravity does the same amount of work and forces with that characteristic are called conservative forces and can have a potential energy associated with them. And so the work done by conservative forces is the opposite to the change in potential energy and the work done by the conservative force here... well, my subscripts are confusing because this is c for crane and this is c for conservative so let's give that a different thing, let's call that cr for crane. So this work done by conservative force is negative mg times the displacement upwards and negative because it's directed downwards and the displacement is upwards and so they are in opposite directions and so that makes the work done negative. And so this negative mgd equals negative ΔPE and so those two things are equal to each other as we showed here. So this is something you already know that you know as an object goes up, its gravitational potential energy increases but yeah... that's just a way of explaining it in terms of conservative forces. Okay! And then when we consider this object being pushed sideways on a surface that does have friction, the pushing force equals the friction force while it's moving at constant speed and then I guess the friction force or sorry, the pushing force is stopped or reduced such that the friction force is greater and decelerates the object till it becomes at rest. So this friction force is a non-conservative force meaning it does a different amount of work depending on the path taken so if this box had instead been pushed through this you know, valley here which is a greater total distance than this distance along this flat surface the friction will do a greater amount of work in this case which is to say that it's non-conservative because the work that the friction does depends on the length of path taken from the starting and to the end points. So this is the start point and this is the end point and then you have the flat surface versus a valley in between, which is longer, you will have different amounts of work done by the friction in both those cases so it's non-conservative. So there's no conservative force here and so the work done by conservative forces is 0 and so the change in potential energy is 0 and this is the big difference between the crane scenario and the box along a surface with friction scenario is that in the crane scenario, there is a gain in potential energy whereas in the scenario with friction, there's no change in potential energy.