Question
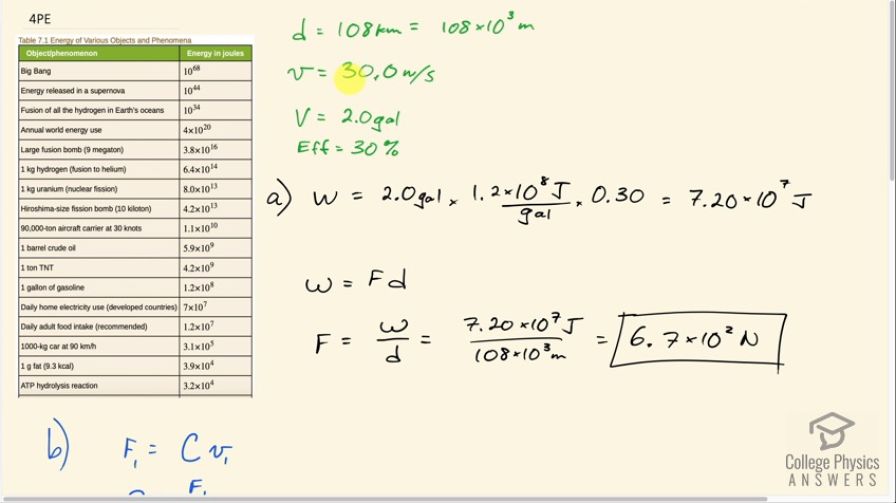
Suppose a car travels 108 km at a speed of 30.0 m/s, and uses 2.0 gal of gasoline. Only 30% of the gasoline goes into useful work by the force that keeps the car moving at constant speed despite friction. (See Table 7.1 for the energy content of gasoline.) (a) What is the magnitude of the force exerted to keep the car moving at constant speed? (b) If the required force is directly proportional to speed, how many gallons will be used to drive 108 km at a speed of 28.0 m/s?
Final Answer
- 1.9 gal
Solution video
OpenStax College Physics for AP® Courses, Chapter 7, Problem 4 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. There's a car traveling 108 kilometers which we convert into meters by multiplying by 10 to the 3; it's traveling at 30.0 meters per second and it uses 2.0 gallons of gasoline and it has an efficiency of 30%. So the energy or the work done, I should say, is the 2.0 gallons of gasoline multiplied by the energy density of gas which we look up in table [7.1]— that is 1.2 times 10 to the 8 joules in every gallon of gasoline— and then we multiply that by the efficiency, written as a decimal, of 0.30 and that is 7.20 times 10 to the 7 joules is the work done by the car. This work done is the force that it's applying against friction times the distance it travels and so we can calculate this force by dividing both sides by the distance. So that's the work done—7.20 times 10 to the 7 joules— divided by the distance—108 times 10 to the 3 meters— which is 6.7 times 10 to the 2 newtons. In part (b), we are told that the force that the car applies is proportional to its speed. So that means there's some constant multiplied by the speed in order to calculate the force and we don't know what this constant is— and it doesn't matter— it's just important to know that one exists. And we can solve for it by dividing both sides by v 1 and we get that the constant is F 1 over v 1 and then that same constant can be used when we calculate this force in the second case when the car is slowed down to 28 meters per second— it would be the same constant multiplied by its new speed— and then we can replace C with this F 1 over v 1 that we had before. By the way we are calculating the amount of gasoline that's gonna be consumed at this new speed but we have to figure out how... you know, we are told that the force is proportional to speed and then we see how that impacts the work calculation and then from the work, we'll figure out how to calculate the volume of fuel consumed. Okay! So the force in the second case then is F 1 over v 1 times v 2 because F 1 over v 1 is this constant of proportionality connecting the force and the speed. So the work done in the second case is this force in the second case times the distance and the distance does not get a subscript because it's the same distance in both cases but we can replace F 2 with this whole thing: F 1v 2 over v 1. Now F 1 times the distance is the work done in the first case so we replace that with w 1 and then now we have connected the two different works together so the work done in the second case is gonna be v 2 over v 1 times the work done in the first case and then we connect work with volume of gas consumed. So the work done is the volume of gas multiplied by the energy density which is usually this letter U multiplied by efficiency and we can solve for V by dividing both sides by U times efficiency. So the volume of gas consumed in the second case is the work done in the second case divided by the energy density of gasoline times the efficiency but we have an expression for this w 2 and it's v 2 over v 1 times w 1. And then we know that volume, in the first case, could be w 1 over U times efficiency which is all this meaning we can replace all that with the volume of fuel used in the first case and so the volume of fuel used in the second case is the ratio of speeds times the volume used in the first case. So that's 28.0 meters per second— is the new speed— divided by 30.0 meters per second— is the old speed— times 2.0 gallons of gas used at the old speed and that gives 1.9 gallons used now.