Question
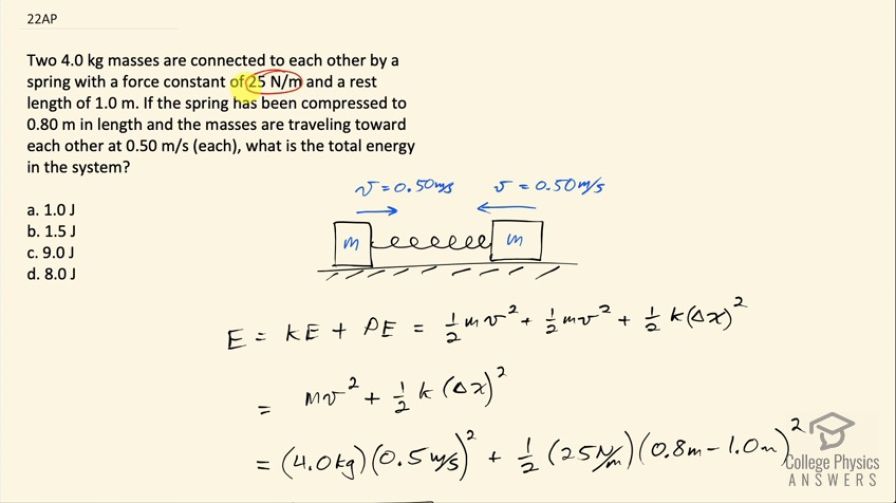
Two 4.0 kg masses are connected to each other by a spring with a force constant of 25 N/m and a rest length of 1.0 m. If the spring has been compressed to 0.80 m in length and the masses are traveling toward each other at 0.50 m/s (each), what is the total energy in the system?
- 1.0 J
- 1.5 J
- 9.0 J
- 8.0 J
Final Answer
(b)
Solution video
OpenStax College Physics for AP® Courses, Chapter 7, Problem 22 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. These two masses on a frictionless surface we assume are connected by a spring with a given spring constant and this spring has been compressed to a length of 0.80 meters compared to its original rest length of 1.0 meter and each mass is moving at 0.50 meters per second and the question is what is the total energy of this system? So that's gonna consist of its total kinetic energy plus the potential energy. The potential energy is this energy in the spring— elastic potential energy— which has a formula one-half times the spring constant times the amount of compression or stretch relative to rest length squared and then we add to that the kinetic energy of each mass each of which is one-half times m times v squared and there's no need for subscripts to distinguish between them because they both have the same magnitude velocity or the same speed, in other words, and they both have the same mass m and same speed v. So one-half mv squared plus one-half mv squared is just mv squared and now we can plug in numbers and we have 4.0 kilograms times 0.50 meters per second squared plus one-half times 25 newtons per meter— spring constant— times a length currently of 0.8 meters for the spring minus its 1.0 meter—rest length— so compression of 0.2 meters, in other words, squared and this is 1.5 joules in total for the energy of the system so the answer is (b).
