Question
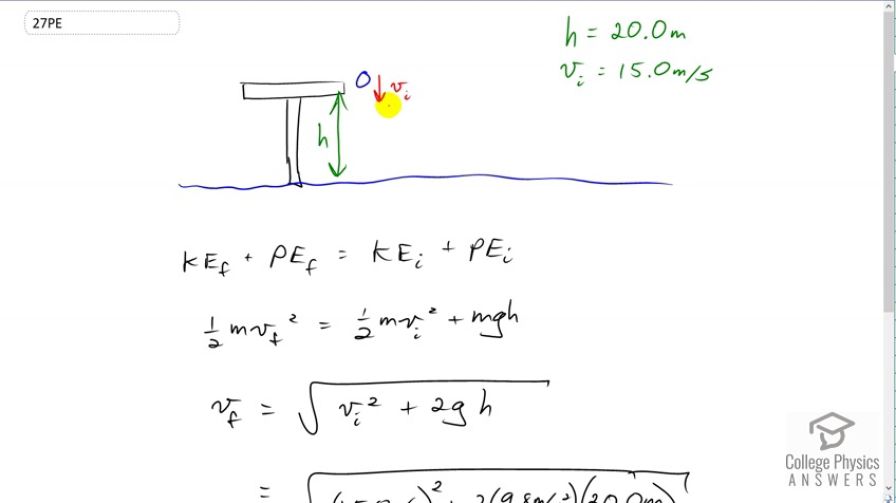
Using energy considerations and assuming negligible air resistance, show that a rock thrown from a bridge 20.0 m above water with an initial speed of 15.0 m/s strikes the water with a speed of 24.8 m/s independent of the direction thrown.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 7, Problem 27 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A rock is thrown down from a bridge towards the water with an initial speed of 15 meters per second at the top, and the bridge is the height 20 meters above the water. We have to find out what the final speed will be of the ball when it reaches this position down here just as it hits the water. So the total final mechanical energy is going to equal the total initial mechanical energy, and we have zero potential energy when it's at the water level because we'll take this to be our reference level. Or we'll say, I don't know, can say h equals zero there and so h represents the height above this reference level. So with a height of zero at the water that means the potential energy is zero. We do have some initial kinetic and potential energies. So let's substitute some formulas for each of these three terms that we have left. We have one half mass times final speed squared equals one half mass times initial speed squared plus the initial potential energy mgh. We can divide both sides by m and multiply both sides by two. Then we get vf equals square root of vi squared plus two gh after we also take the square root of both sides. Substituting in numbers we have square root of 15 meters per second initial speed and then square that, plus two times 9.8 meters per second acceleration due to gravity, times 20 meters height of the bridge, giving us 24.8 meters per second will be the speed of the rock when it hits the water.
