Question
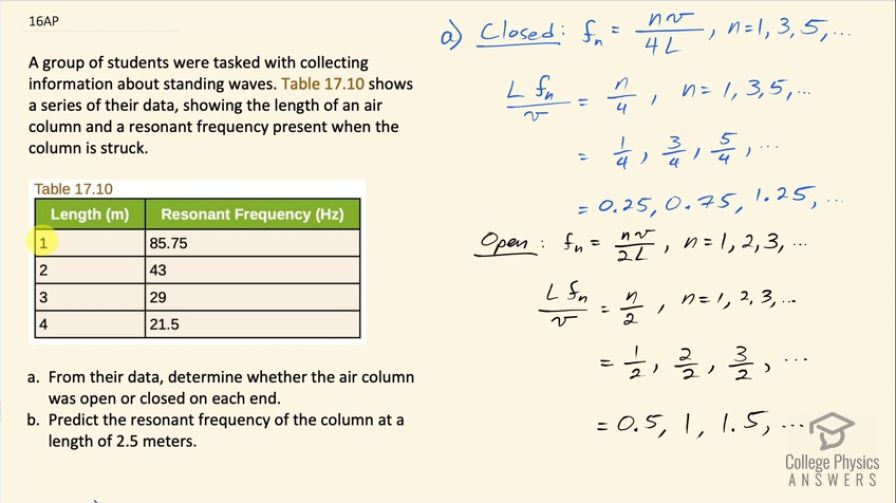
A group of students were tasked with collecting information about standing waves. Table 17.10 a series of their data, showing the length of an air column and a resonant frequency present when the column is struck.
- From their data, determine whether the air column was open or closed on each end.
- Predict the resonant frequency of the column at a length of 2.5 meters.
Final Answer
- Closed
Solution video
OpenStax College Physics for AP® Courses, Chapter 17, Problem 16 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A group of students have collected this data for a resonant frequency in a column of air with this length in meters and we are asked to figure out what kind of air column this is? Whether it's open at both ends or maybe closed at one end? So we have formulas for resonant frequency based on a closed end at one end or open at both ends. So for when the tube is closed at one end, the resonant frequency then is some integer n which could be number 1, 3, 5 and so on times the speed of sound divided by 4 times the length. We can rearrange this to solve for n over 4 and the reason that's useful is because this n over 4 is the thing that distinguishes the formula for closed end versus the formula for a tube open at both ends. So for a tube open at both ends if we multiply both sides by L and divide both sides by v and we'll end up with this line here where we have L times the resonant frequency divided by v is n over 2 whereas for a column closed at one end, multiplying both sides by L over v gives us L times resonant frequency divided by speed of sound equals n over 4 and that could be n over 4 could be one quarter could be three quarter... could be five quarters... we don't know actually know which resonance this is for each length like when there's a column of 1 meter length, we don't know whether this is the fundamental frequency or whether it's some overtone so we don't know what n is in other words but we can list a bunch of possibilities for it and it could be one quarter, it could be three quarters five quarters whereas decimals, it's 0.25, 0.75, 1.25 this is the sequence that's possible if it's closed at one end whereas if it's open at both ends, the possibilities for L times resonant frequency divided by v is one-half, 2 over 2, 3 over 2 or 0.5, 1 and 1.5. So what we are going to do is with a spreadsheet, we are going to figure out what is this as a decimal. So we'll use a Google spreadsheet copying the length and the frequency here and this is frequency times length divided by speed of sound so that's B2 times A2 divided by 343— speed of sound is 343— and you can see that to two decimal places, we are getting 0.25 every time so that is strong confirmation that this is a tube closed at one end so we are getting 0.25 as our answer to L times resonant frequency divided by v and 0.25 is not possible given open at both ends... only 0.5 is the smallest number possible so we can say for sure, this is a tube closed at one end. Now it's asking us to predict the resonant frequency if the column had a length of 2.5 meters so now that we know the formula is nv over 4L because it's closed at one end with n 1, 3 or 5, we can figure out the fundamental frequency for example and that will be 1 times 343 divided by 4 times 2.5 meters and that is 34.3 hertz.
