Question
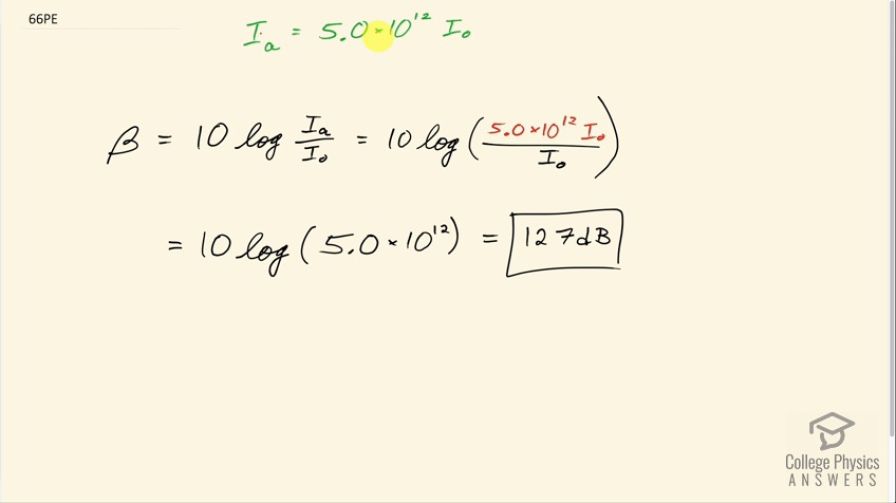
If a woman needs an amplification of times the threshold intensity to enable her to hear at all frequencies, what is her overall hearing loss in dB? Note that smaller amplification is appropriate for more intense sounds to avoid further damage to her hearing from levels above 90 dB.
Final Answer
127 dB
Note: this seem unrealistic. This kind of amplification would further damage the woman's hearing. The question should be modified for a much lower ratio of sound intensity.
Solution video
OpenStax College Physics for AP® Courses, Chapter 17, Problem 66 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A woman's hearing is damaged such that she needs an intensity that's amplified to 5.0 times 10 to the 12 times the intensity at the normal threshold of hearing. So the amount by which she needs to have her amplification in decibels will be 10 times logarithm of the amplified intensity divided by the threshold of hearing intensity. So that's 10 times log of 5.0 times 10 to the 12 I naught over I naught and the I naught's cancel so we have 10 times log of 5.0 times 10 to the 12 which is 127 decibels. Now I'm not sure that the question is quite reasonable because this is a huge decibel increase which would damage hearing quickly. So I think this 10 to the 12 is probably a mistake in the question.
