Question
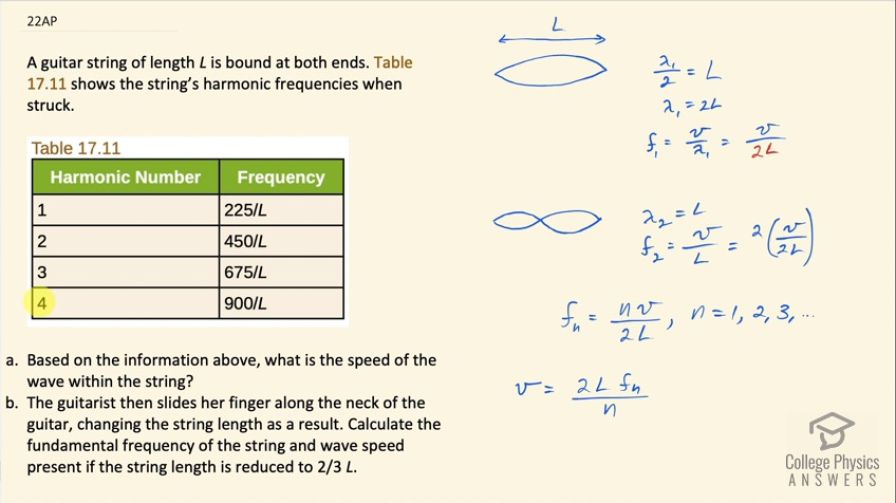
A guitar string of length L is bound at both ends. Table 17.11 shows the string’s harmonic frequencies when struck.
- Based on the information above, what is the speed of the wave within the string?
- The guitarist then slides her finger along the neck of the guitar, changing the string length as a result. Calculate the fundamental frequency of the string and wave speed present if the string length is reduced to .
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 17, Problem 22 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A guitar string of length L is attached at both ends and we have this data table, which tells us the harmonic number and the frequency of that harmonic and these are all in terms of dividing by L. So we need to find a formula to figure out what is the speed of the wave on a string because that's question (a) and if we draw a picture of the fundamental, where we have a node at both ends because that's where it's attached and the single antinode midway between those nodes, this is a picture showing half of a wavelength so half of the fundamental wavelength— and that's with the subscript 1 there represents it's the first harmonic, which is also known as the fundamental— that equals the length L and we can solve for λ 1 and it's going to be 2L and then find the frequency and if we can show a pattern for the frequency then we can solve that frequency formula for v because frequency will be in terms of v, it will be the speed divided by the wavelength because the wave equation is that the speed of a wave is its frequency multiplied by its wavelength so the frequency can be written this way after you divide both sides by λ here and then this wavelength is 2L in the case of the fundamental. For the second harmonic, we'll have a node midway between the two ends and this shows a full complete wavelength so wavelength for the second harmonic is L in that case, f 2 is v over L and we can write this in terms of f 1 in order to notice a pattern and so we can write this as 2 times v over 2L and because 2 times v over 2L will become v over L and this is 2 times f 1 and we see that we are going to multiplying by an integer that increases by 1 as we consider each harmonic... each subsequent harmonic. So the nth harmonic then is going to be this natural number n times the wave speed divided by 2L. Okay and then this can be solved for v by multiplying both sides by 2L over n and then we have this formula for the wave speed: 2L times the frequency divided by n and this analysis follow the same pattern as a tube that was open at both ends by the way so whereas here we have a node at both ends, a tube that's open at both ends has an antinode at both ends and the formulas are the same. Okay! So this is... we can't just plug... well, numbers into this because we don't know the length L but we do know the frequency as a fraction of L and so we can multiply them together and that will make this numerator and we have a spreadsheet that's going to give us the wave speed for each harmonic and then we'll take the average to get our answer. So here's a Google spreadsheet here where we have the data table information copied here this column I created—it's the frequency times L because that's part of this formula... we have frequency times L so this column here represents that— frequency times L and it's just going to be the numerator because 225 divided by L times L is 225 and likewise for the rest of these rows and the wave speed is going to be 2 divided by the harmonic number n times this product of L and frequency and so this is the frequency times L multiplied by 2 divided by the harmonic number, which is n and this next one is the same formula but just looking at row 3 and then row 4 and so on. We need the same wave speed in every case—450—so the average of four instances of 450 is clearly 450 so that's going to be our answer. Okay let's write it down then right away so v equals 450 meters per second... there we go! Part (b) says that the guitarist then slides her finger along the neck of the guitar changing the string length and we have to calculate the fundamental frequency now and the wave speed given that the string length is two-thirds of what it was before. So a formula for the nth harmonic in this new case so I'll denote everything that's new with a prime on it is gonna be the harmonic number times the new wave speed v prime divided by 2 times the new length L prime and this is a formula that we have established before and we are just reusing it but with new values for part (b). However the wave speed is going to be the same as it was before because wave speed in a string is the square root of the tension in the string divided by its mass per length and you know, even though the length has changed, the mass of the vibrating portion has changed in the same proportion so this fraction m over l is the same— we assume that the string has mass evenly distributed or uniformly distributed along this length— in which case, the mass per length is the same regardless of the length itself. I looked this formula up in a different textbook actually and I don't know how you are meant to know this from the OpenStax textbook or maybe I missed it in there somewhere or you know, I guess you could Google it too. So this means that v prime is 450 meters per second so we can plug v in for v prime. The length L prime is two-thirds L and so we can substitute that in here and then multiply well, you know, dividing by two-thirds is the same as multiplying by 3 over 2 so we have 3 over 2 times nv over 2L and nv over 2L is f n—the frequency of the nth harmonic from part (a)— and so the frequency of the fundamental in this new case is going to be 3 over 2 times the fundamental before. So that's 3 over 2 times 225 over L and that is 338 over L.
