Question
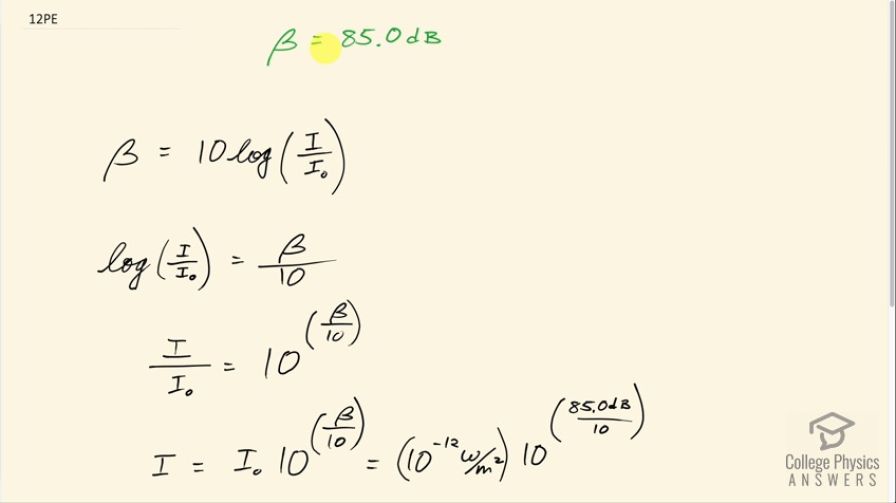
What is the intensity in watts per meter squared of 85.0-dB sound?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 17, Problem 12 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Given a sound intensity level of 85.0 decibels, the question is what is the intensity of the sound in watts per square meter? So the sound intensity level, β, equals 10 times the logarithm of the intensity divided by some reference intensity which is the threshold of hearing for a person with normal hearing and this is the base 10 logarithm which we don't have to write because the assumption is when you see the word log, it means base 10 log. So we'll divide both sides by 10 here and then switch the sides around so we have logarithm of intensity divided by reference intensity equals sound intensity level divided by 10. and then make both sides exponents for 10 because when you do that 10 to the power of logarithm of base 10 is going to be just the argument of the logarithm because the logarithm tells you what exponent does 10 need in order to equal the thing that you are taking the log of and so since the logarithm is gonna give that exponent and we have 10 as our base, 10 to this exponent is going to be the argument of the log so we have I over I naught here. And on the right hand side, we make this an exponent for 10 and we write 10 to the power of β over 10. Then we multiply both sides by I naught and you get the intensity then is the reference intensity times 10 to the power of the sound intensity level over 10. So it's 10 to the minus 12 watts per square meter— that's the threshold of hearing— times 10 to the 85.0 decibels over 10 and that is 3.16 times 10 to the minus 4 watts per square meter.
