Question
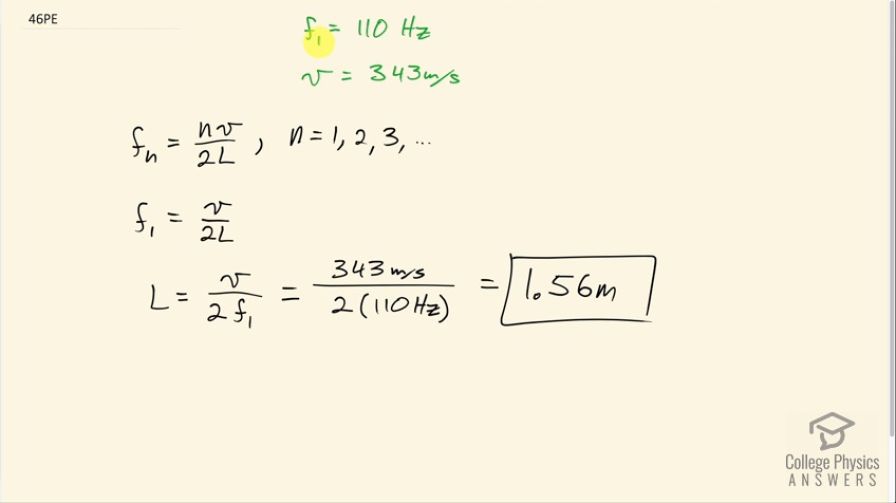
What length should an oboe have to produce a fundamental frequency of 110 Hz on a day when the speed of sound is 343 m/s? It is open at both ends.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 17, Problem 46 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. An oboe which can be modeled as a tube that's open at both ends is meant to have a fundamental frequency which we'll label f 1 of 110 hertz on a day when the speed of sound is 343 meters per second. So the formula for the nth harmonic for a tube open at both ends is n times the speed of sound divided by 2 times the length of the tube where n is a natural number 1, 2, 3 and so on. That means f 1 being the fundamental is 1 times v so we replace the letter n with number 1 times the speed of sound over 2L and we can solve for the length by multiplying both sides by L over f 1 and this gives us L is v over 2f 1. So that's 343 meters per second divided by 2 times 110 hertz which is 1.56 meters and that's the length the oboe would need.
