OpenStax College Physics for AP® Courses, Chapter 17, Problem 25 (Problems & Exercises)

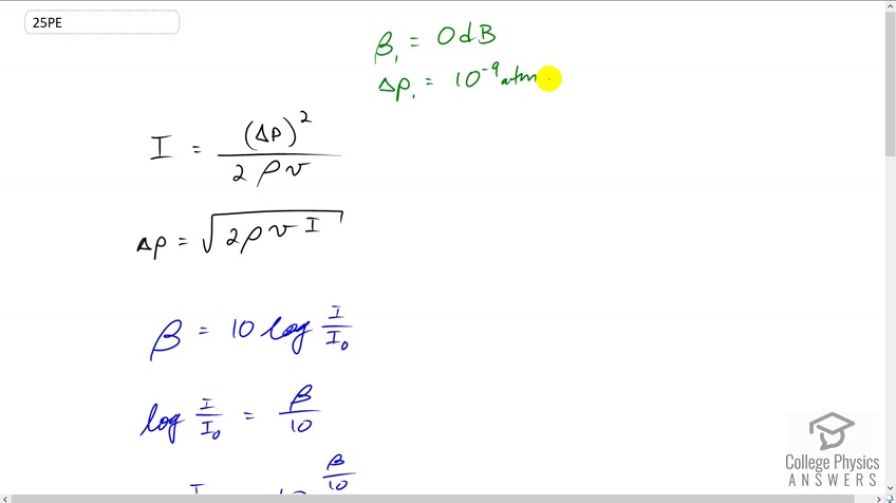
This is College Physics Answers with Shaun Dychko. We are told that sound that has a sound level of zero decibel has a pressure amplitude of ten to the minus nine atmospheres and we are asked you know given a sound level of 60 decibels in per day what would the pressure amplitude be then or in part b, the sound level of 120 decibels, yep. What would be the sound level be in that case? So, we need to find an expression for pressure amplitude in terms of the original pressure amplitude and the different sound level. Now, we know that the intensity of a sound is the pressure amplitude squared divided by two times the density of the medium that the sound is travelling through, air in this case, times the speed of sound in air and we will solve this for delta p and we will multiply both sides by two Roh v and then take square root of both sides to get this expression. So, that’s the expression of pressure amplitudes in terms of the intensity. We don’t know what the intensities are! We know what the sound level is and so we need to substitute for I and replace it to something containing beta. So beta, the sound level is ten times logarithm of intensity divided by some reference intensity and we will solve this for I. We are gonna divide both sides by ten, switch the sides around to get the unknown on the left and then make both sides power ten. On the left sides we are left with I over I naught and on the right side we have ten to the power of beta over ten and then multiply both sides by I naught and we are left with intensity is with reference intensity times ten to the power sound level divided by ten. So, the pressure amplitude then is equal to square root, two roh v times all of this substituted in place of I. So, I have written that in red here. So, its I naught ten to the power beta over ten and now we are doing with two different pressure amplitudes, we are given, Delta P one and we are going to find Delta P two. So, lets take ratio of those two pressure amplitudes. So, that's gonna be square root of two Roh v I naught ten to the power Beta two over ten divided by square root of two Roh v I naught ten to the power Beta one over ten. Now, the nice thing happens here is these factors of square root two Roh v cancels and I naught as well and we are left with square root of ten to the power of Beta two over ten divided by ten to the power Beta one over ten. Now when you are dividing two powers with the same base, you can just take the difference between the exponents and so we have ten to the power Beta two over ten minus Beta one over ten and that’s a common denominator there which is convenient so we have square root of ten to the power Beta two minus Beta one all over ten. Now, this can be made to look a little bit cleaner still, I would write this square root as exponent, one half and then when you have a power to a power, you can multiply the exponent and so Beta two minus Beta one over ten times a half is beta two minus beta one over twenty, ten times two. So, at long last we now have a formula for the sound pressure amplitude in the seconds case as a function of pressure amplitude in the first case and the different sound levels and we will multiply both sides with Delta p one. So, the pressure amplitude in the second case is pressure amplitude in first case times ten to the power of different sound levels divided by 20. So, we are told in first case that the pressure amplitude at zero decibels is ten to the minus nine atmospheres and we will multiply that by ten to the power of 60 decibels in the second case minus zero decibels over 20 and this ten to the minus six atmospheres is the sound pressure level in the second case on 60 decibels and then for part b we substitute 120 decibels in place for the sound level in case two and this works out to ten to the minus three atmospheres.