Question
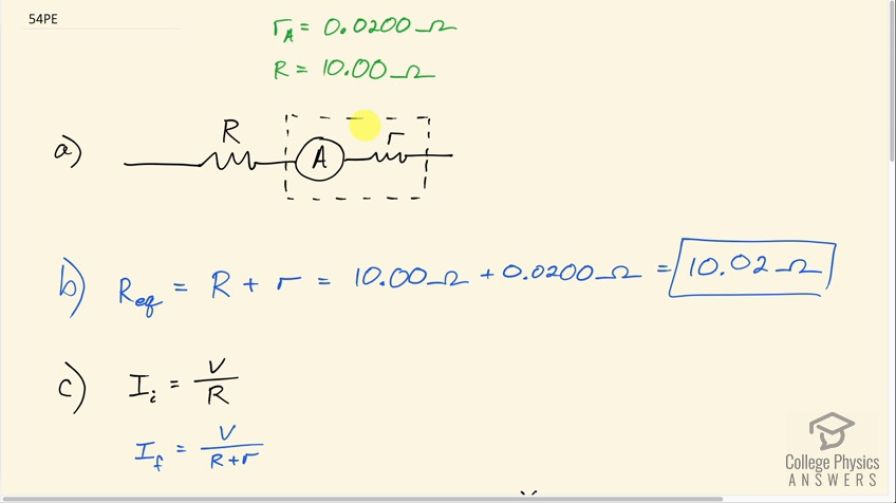
A ammeter is placed in series with a resistor in a circuit. (a) Draw a circuit diagram of
the connection. (b) Calculate the resistance of the combination. (c) If the voltage is kept the same across the combination as it was through the resistor alone, what is the percent decrease in current? (d) If the current is kept the same through the combination as it was
through the resistor alone, what is the percent increase in voltage? (e) Are the changes found in parts (c) and (d)
significant? Discuss.
Final Answer
- Please see the solution video.
- These changes are not significant since they are less than 0.5% which is likely less than the uncertainty in the measurements.
Solution video
OpenStax College Physics for AP® Courses, Chapter 21, Problem 54 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. An ammeter has an internal resistance of 0.0200 ohms and it's drawn in a circuit diagram like this; we don't have that other ammeter diagram that you might be familiar with which looks like this this is the internal resistance of the galvanometer and a shunt resistance down here all of this is combined into this single thing here where this resistance here represents the equivalent resistance of these two combined maybe I should give it a different... I will call it r prime say, it's not the same as the internal resistance of the galvanometer, it's the parallel combination of R plus r I mean R prime is R reciprocal plus internal resistance of the galvanometer reciprocal all to the power of negative one this is to add these two resistances in parallel to get this r prime, which is this resistance here given to us... oh, r subscript A that's what I called it yeah, r A for ammeter. Good! Okay! This other resistance put in series with it is 10.00 ohms and there's the circuit diagram; part (b) says what is the total resistance of this combination? So we have this resistance external to the ammeter plus this resistance of the ammeter—r A— so that's 10.00 ohms plus 0.0200 ohms and that's 10.02 ohms in total. Okay... let's put a little subscript A in every single little r we see here just to be clear that that's the total resistance of the ammeter. Alright! Part (c) it says if the voltage is kept the same across this combination as it was through the 10.00 ohm resistor alone, what is the percent decrease in current? Okay. So initially we have just this resistance R by itself and there's some voltage across this resistor and we will call it V and there's some initial current going through this resistor and so that initial current is going to be the voltage divided by the resistance; this is a rearrangement of Ohm's law— V equals I initial times R— it's solving for I initial by dividing both sides by R. Okay! And then the final scenario is where you have this resistance in combination with the ammeter and it's gonna have the same voltage we are told same voltage so voltage is being measured like this. So the V does not get a subscript— it's the same voltage in both cases— but we have current final, it's a different current now because there's a different resistance here between the terminals of the voltmeter and so this resistance then is this total R plus the resistance of the ammeter and what is the percent change in the current then? So the percent change will be the final current minus the initial current divided by the initial current times a hundred percent. So the final current is this written here minus V over R for initial current all divided by V over R times 100 percent. So we'll divide every single term by V so that makes 1 over R plus r A minus 1 over R all over 1 over R and then multiply top and bottom by R as well basically, we are multiplying top and bottom by R over V every single term is being multiplied by R over V and then on the bottom, we are left with 1 so I am not gonna bother writing that and on the top, we are left with R over R plus r A minus this becomes 1 times a 100 percent. So that's 10.00 ohms divided by this total resistance of the two things combined which is 10.02 ohms— as we found in part (b)— minus 1 times 100 percent is negative 0.1996 percent. So the current has decreased—that's what the negative sign means— has decreased by about 0.2 percent as a result of this ammeter being put in series with this resistor and we expected a decrease in current because given a constant voltage, we have increased the resistance here and so when you have current equals voltage divided by resistance in general as you increase this denominator holding the numerator constant, we would expect this quotient to reduce. Okay! Part (d) says the current is kept the same through the combination as it was through the 10.00 ohm resistor alone, what is the percent increase in voltage? So now we are going to change the voltage so that the current is constant... let's copy this over here... and write it let's say right here approximately that didn't go where I wanted it but let's put it where it belongs. Good... okay! Now we are going to make the current constant so the same current in both cases but now their voltage will change this is V initial and V final. So V initial is current multiplied by this resistor on its own and V final is gonna be the same current multiplied by this resistance plus the resistance of the ammeter and the percent difference will be V f minus V i over V i times 100 percent so substituting for these each V term, we have current times total resistance minus current times this resistor by itself divided by IR times 100 percent. So dividing both these terms in the numerator by the denominator gives us R plus r A over R minus 1 times 100 percent. So that's 10.02 ohms divided by 10.00 ohms minus 1 times 100 percent is 0.2000 percent and as expected, we are having an increase in voltage in order to maintain the same current through this now larger total resistance. But neither of these changes are significant since they are less than about 0.5 percent, which is likely less than the uncertainty in the measuring device anyway.
