Question
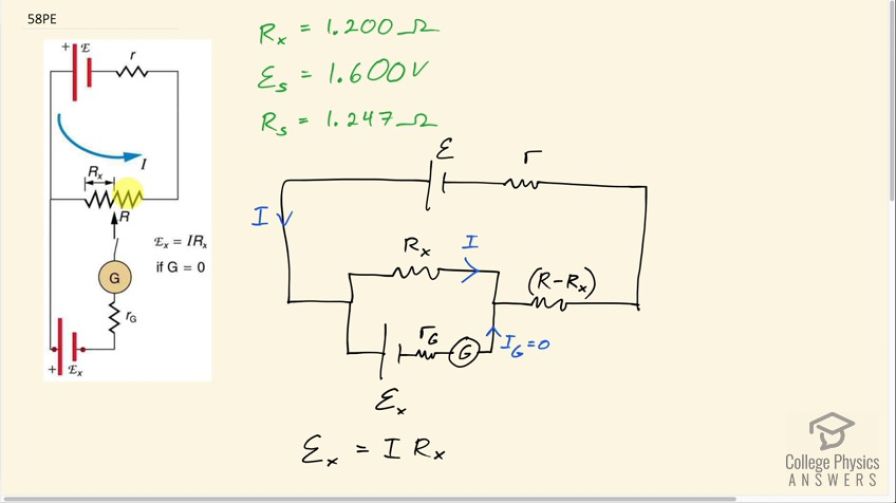
Calculate the of a dry cell for which a
potentiometer is balanced when , while an alkaline standard cell with an emf of 1.600 V requires to balance the potentiometer.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 21, Problem 58 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This is a circuit diagram for potentiometer I have drawn it slightly differently than the way it's drawn here but it's the same thing; we have this resistance R x which is varied such that the current through this galvanometer or the current through this branch containing the unknown emf ε x is zero. And when that happens, we know that the potential is only that of this unknown emf; there's no drop across any internal resistance in it or anything because there's no current through it at all and so it's a direct measurement of the emf and since this and this are in parallel, the potentials are the same and so if only we knew what this current I was then we can multiply that by this known R x and then figure out what the potential is across this branch and then that therefore is the same as the potential across the branch with the ε x. So we figure out what that current is by replacing this emf with a known emf a standard, letter s I think stands for 'standard' some known emf and then adjust the resistance such that the current is zero through this standard emf and then this R s for standard is known and then we can figure out this current I from that because the current across this branch will be the same as the current across this known emf, which we know is ε s and that equals the current times R s and then we can solve for I by dividing both sides by R s and then this current is going to be substituted in here to figure out what emf x is and we do that here. So the unknown emf is the standard emf divided by the standard resistance multiplied by the resistance when the unknown emf is in that circuit and this works out to 1.600 volts divided by 1.247 ohms multiplied by 1.200 ohms and that is 1.540 volts is the unknown emf.
