Question
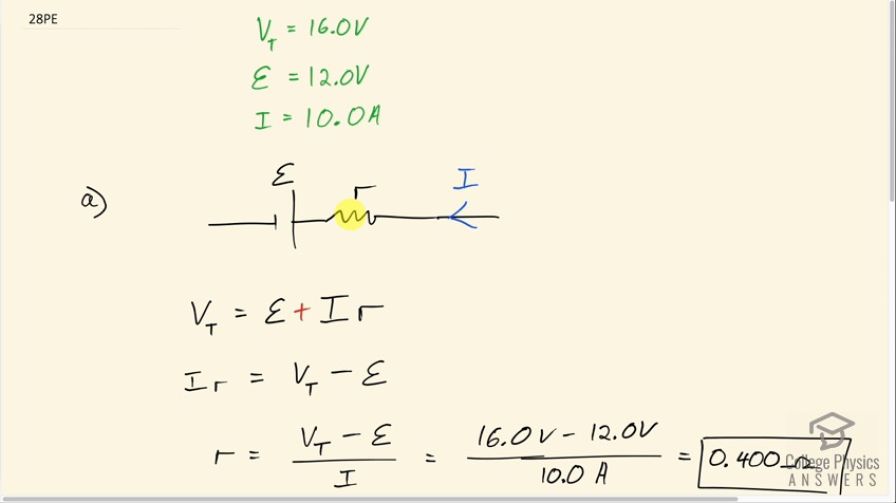
A 12.0-V emf automobile battery has a terminal voltage of 16.0 V when being charged by a current of 10.0 A. (a) What is the battery’s internal resistance? (b) What power is dissipated inside the battery? (c) At what rate (in ) will its temperature increase if its mass is 20.0 kg and it has a specific heat of , assuming no heat escapes?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 21, Problem 28 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A car battery with a 12.0 volt emf is being charged by a 10.0 amp current and when that happens, it has a terminal voltage between here and here of 16.0 volts. So since the current is charging the battery, we know the direction of the current is to the left in this picture; normally when we have a circuit with the battery providing the current, we have the current coming out of the positive terminal but in this case because the battery is being charged, there's some external source of current that is driving a current backwards through the battery. So in that case the terminal voltage then is the emf plus the current times the internal resistance and that plus comes from thinking about Kirchhoff's Loop rule; if we were to traverse this section, you know, of an imaginary loop we don't know what the rest of the circuit is in the motor and it doesn't matter but just consider starting here at one terminal of the battery and move to the right, we start from the negative terminal and go to the positive terminal— that's an increase in potential— and then going across the resistor if we are traversing to the right, well, the current is going to the left, this is going to be a positive change in potential when traversing a resistor in the opposite direction to the current, you put a plus sign in front of that potential change. So we can rearrange this then to figure out what the internal resistance is. We'll begin by subtracting emf from both sides and we have current times internal resistance is the terminal voltage minus the emf and then divide both sides by the current and so the internal resistance of the terminal voltage minus the emf divided by the current. So that's 16.0 volts minus 12.0 volts divided by 10.0 amps, which is 0.400 ohms. Part (b) asks what power is being dissipated in the battery? And so it's being dissipated by this internal resistance and so we take the current squared multiplied by that internal resistance so that's 10.0 amps squared times 0.400 ohms, which is 40.0 watts. Part (c) asks assuming no heat is escaping from the battery and there's heat being produced at this rate of 40.0 watts, what will be the rate of temperature increase of the battery given that it has a mass of 20.0 kilograms and a specific heat of 0.300 kilocalories per kilogram per Celsius degree? Okay! So power is the rate at which heat is being produced in this case and heat we have a formula, which is mass times specific heat times change in temperature and we can just consider this part as our answer; this is gonna be the rate of temperature increase—Δ temperature over time— and we can solve for it by dividing both sides by mc or multiplying by 1 over mc and so the rate of change in temperature then is the power divided by the mass times its specific heat. So that's 40.0 watts but I have written watts in units of joules per second just so we can see how to get our answer in degrees Celsius per minute, which is what the questions asks for. So we have 40.0 joules per second divided by 20.0 kilograms times 0.300 kilocalories per kilogram per Celsius degree and this kilocalories is not an mks unit— it is not a meters, kilograms or seconds type unit; it's not a SI unit so to speak— and so we will convert it into joules, which is by multiplying by 4184 joules per kilocalorie and these joules cancel here and these kilograms cancel and this Celsius degree being in the denominator of a denominator ends up in the numerator here. So we have 0.00159337 Celsius degrees per second is the rate of temperature increase. Now you can see why these seconds are handy here because we can see to convert into Celsius degrees per minute, we multiply it by 60 seconds per minute and this works out to 0.0956 Celsius degrees per minute is the rate of temperature increase of this battery.

