Question
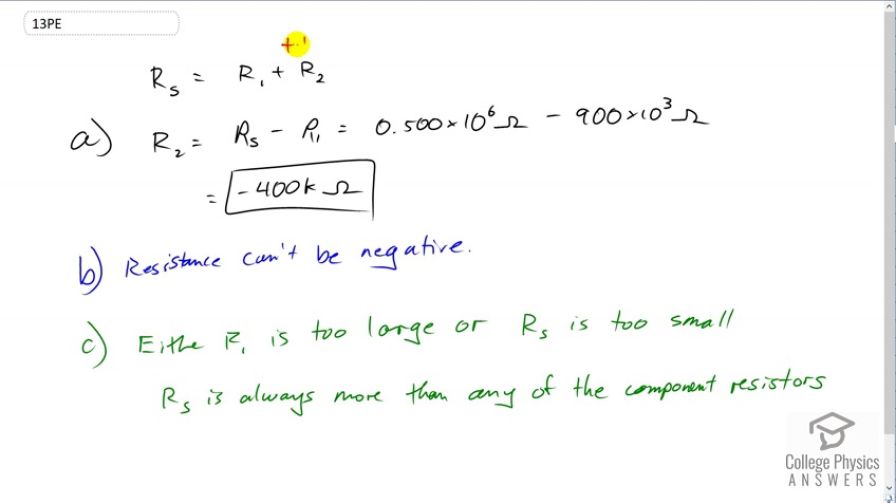
Two resistors, one having a resistance of , are connected in series to produce a total resistance of . (a) What is the value of the second resistance? (b) What is unreasonable about this result? (c) Which assumptions are unreasonable or inconsistent?
Final Answer
- Resistance can't be negative
- Either is too large, or is too small. is always more than any of the component resistors in series.
Solution video
OpenStax College Physics for AP® Courses, Chapter 21, Problem 13 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We are told that two resistors are connected in series and we have to solve for R2. And so we’ll subtract R1 from both sides and we get R2 is the total resistance in series minus R1. So that’s 0.5 Mega Ohms. Mega is the prefix meaning multiplied by ten to the six. And then we have minus 900 kilo Ohms. Kilo is the metric prefix meaning multiplied by ten to the three. And so this works out to negative 400 kilo Ohms. And that’s not reasonable because resistances can’t be negative. And so either R1 is too large and so we shouldn’t be subtracting so much away from this total. And it might look like this number is smaller than this one because this is only ten to the three whereas this is ten to the six. I haven’t technically written this in scientific notation. For scientific notation would say: it would say don’t have these zeros that’s for sure. And one number to the left of the decimals, so this would be 5.00 times ten to the five. And this would be 9.00 times ten to the five. Now it’s easier to compare because they have the same multiplier here. And so this clearly is bigger and with that we can compare the nine to the five and nine is bigger than five. So, either R1 is been given to us as a number that is mistakenly too large. Or the series resistance that we’re given is too small. Maybe this number should in fact be much bigger, because the total resistance of two resistors in series is always more than any one of the particular resistors.
