Question
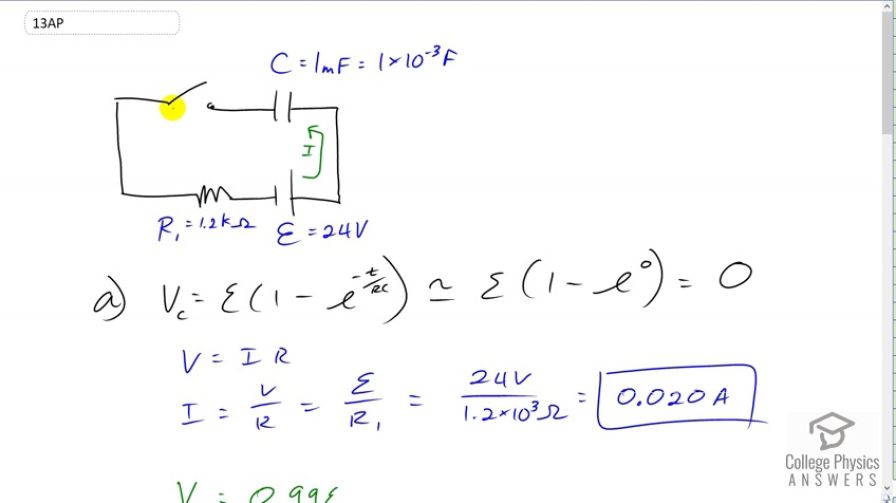
An uncharged capacitor is connected in series (with a switch) to a resistor and a voltage source . Assume , and .
- What will be the current through the circuit as the switch is closed? Draw a circuit diagram and show the direction of current after the switch is closed. How long will it take for the capacitor to be 99% charged?
- After full charging, this capacitor is connected in series to another resistor, . What will be the current in the circuit as soon as it’s connected? Draw a circuit diagram and show the direction of current. How long will it take for the capacitor voltage to reach 3.24 V?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 21, Problem 13 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
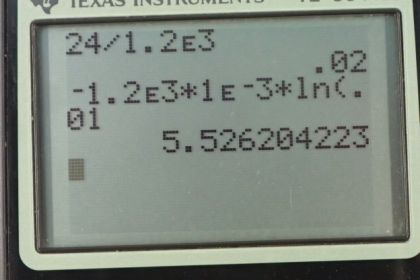
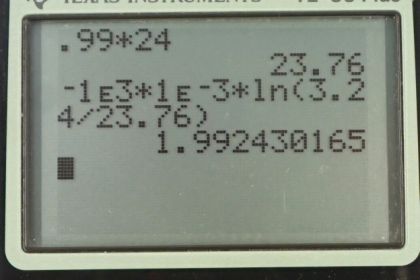
This is College Physics Answers with Shaun Dychko. We have a circuit with a 1.2 kilo Ohms resistor, an EMF with a 24 Volts and a capacitor of milliFarads, and a switch that is initially open, and the capacitor is uncharged. So the question in part a asks us just at the moment when the switch is closed what will be the current through the circuit. And so, well, the voltage across the capacitor is one concern and that equals EMF times one minus e to the negative t over the time constant resistance times capacitance. But, since this is just at the very instant when the switch is closed, the time that has passed is essentially zero. So this becomes one to minus e to the zero is one. One minus one is zero. So the voltage across the capacitor at the beginning is zero. And so we can pretend that it’s not there. Then we can just imagine that there’s just a wire there from the very instant at the beginning. In which case, this is a regular Rs. Just a circuit with a resistor and an EMF in it. So we can say V equals IR. And so the current is going to be the voltage divided by resistance. The voltage is the EMF here divided by R1, which is 24 Volts divided by 1.2 times ten to the three Ohms which is 0.020 Amps. And after a while, there will be a voltage across the capacitor. And we’re asked to find out what time will the voltage across the capacitor be in 99 percent of the EMF voltage. And so we’ll substitute 0.99 epsilon in place of Vc. And then we could divide both sides by epsilon. And after switching the sides around, one minus e over t over Rc equals 0.99. And then subtract one from both sides. And then you get negative 0.1 here. Then, multiply both sides by negative one to the positive e to the negative t over Rc equals positive 0.01. Then take the natural log rhythm of both sides. And on the left, you end up with negative t over Rc and on the right, you have natural log and 0.01. Then, multiply both sides by negative Rc. And you get the time is negative Rc times natural log of 0.01. And then substitute numbers. We have negative 1.2 times ten to the three Ohms times one times ten to the minus three Farads times natural log of 0.01, which is 5.5 seconds. And so after 5.5 seconds the capacitor will be charged to 99 percent of the EMF. Then we are told that the capacitor is removed from that circuit and put into a different one that has only a single resistor R2 which is one kilo ohm. And how long will it take for the capacitor voltage to be reduced to 3.24 Volts. So, the voltage across the discharging capacitor is its initial voltage times e to the negative t over resistance times capacitance. And we can divide both sides by V naught. And we got e to the negative t over Rc equals V over V naught. And then take the natural log of both sides. And we get negative t over Rc equals natural log rhythm of V over V naught. And then multiply both sides by negative Rc. And you get t as negative Rc times natural log of V over V naught. So the initial voltage V naught is 99 percent of epsilon so that’s 0.99 times 24 Volts which is 23.76 Volts. And the final voltage we’re told is 3.24. And so we have time is negative one times to ten to the three ohms for R times one times ten to the minus three Farads for C times natural log rhythm 3.24 Volts for V divided 23.76 Volts for V naught giving us 2.0 seconds.