Question
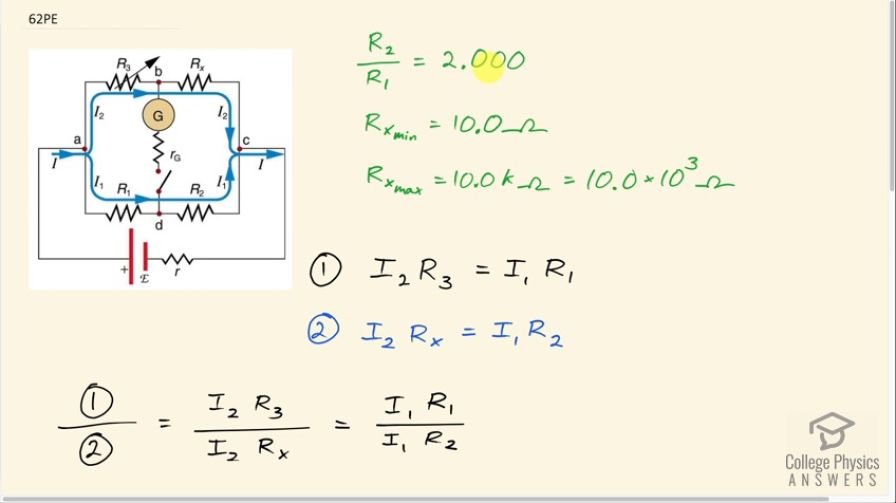
Suppose you want to measure resistances in the range from to using a Wheatstone bridge that has . Over what range should be adjustable?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 21, Problem 62 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. We have a Wheatstone bridge in which the ratio of resistance two to resistance one is 2.000 and we want to be able to put resistances here for R x that are in the range of 10.0 ohms up to 10.0 kiloohms, which is 10.0 times 10 to the 3 ohms and given all that's true, what resistance should be possible for R 3? So R 3 is a variable resistor and it should be able to vary between what extremes? Well, we are gonna solve for R 3 in the two scenarios where we have R x minimum of 10 and then R x maximum of 10.0 kiloohms so we are gonna solve for R 3 twice. We'll figure that out by deriving an equation for it by first noting that since there's no current through this galvanometer—and this switch is closed by the way— that means potential at point b is the same as potential at point d in which case the potential between a and b is the same as between a and d because well this side of both these resistors has to be the same potential because they are connected and then this side of both resistors is also the same since they are essentially connected and there's no current flowing here. So I 2 times R 3, which is the potential drop across R 3, is the same as I 1R 1, which is the potential drop across this resistor. And then since this end of R x and R 2 are both at the same potential at point c and the other end of both resistors are at the same potential at points b and d, we can say that the potential drop across R x and the potential drop across R 2 are also equal and in terms of Ohm's law, we can say the potential drop across R x is I 2R x and across R 2 is I 2R 2. So then we can divide these two equations to figure out what R 3 is. So equation one divided by equation two is left sides are I 2R 3 divided by I 2R x and then the right side is I 1R 1 divided by I 1R 2 and the I 2's cancel and so do the I 1's and we can multiply both sides by R x to solve for R 3 and so R 3 then is R x times R 1 over R 2. So R 3 at its minimum is R x minimum times R 1 over R 2 but we are given R 2 over R 1 which is the reciprocal of R 1 over R 2 so we will write R 1 over R 2, which was given to us and then take the reciprocal of it because that's what we want in our formula. So we have 10.0 ohms at a minimum for R x times 2.000, which is R 2 over R 1 to the power of negative 1, and that gives 5.00 ohms and then R 3 maximum is when R x is at its max that's 10.0 kiloohms times 2.000 to the negative 1 and that's 5.00 times 10 to the 3 ohms. So R 3 needs to be able to vary from 5.00 ohms up to 5.00 times 10 to the 3 ohms.