Question
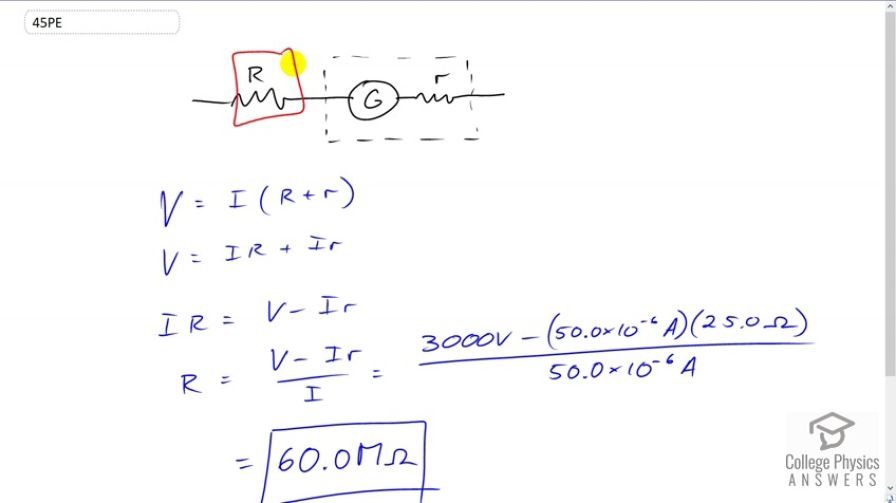
Find the resistance that must be placed in series with a galvanometer having a sensitivity (the same as the one discussed in the text) to allow it to be used as a voltmeter with a 3000-V full-scale reading. Include a circuit diagram with your solution.
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 21, Problem 45 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A voltmeter consists of a galvanometer with an internal resistance combined with some external resistance, you know, external to the galvanometer but internal to the voltmeter. The voltmeter leads would be from here to here. This would be connected to the circuit that's being measured. So the voltage at which full scale deflection occurs is going to equals the sensitivity of the galvanometer, sensitivity being the current through it that causes full scale deflection, multiplied by the total resistance which is these two resistors added together because they're in series, so capital R plus little r, and then we're going to be solving for what is capital R needed such that you have full scale deflection when measuring three thousand volts, given a galvanometer with sensitivity of 50 microamps and an internal resistance of 25 ohms. So distribute the I factor into the brackets there and we have I times capital R plus I times little r and then subtract I little r from both sides, and then divide both sides by capital I -- we're solving for capital R here -- and we get the resistance is the voltage minus current times internal resistance divided by the current. So that's three thousand volts which cause full scale deflection, minus the 50 microamps times the 25 ohms divided by 50 microamps, giving is 60.0 mega ohms.
