Question
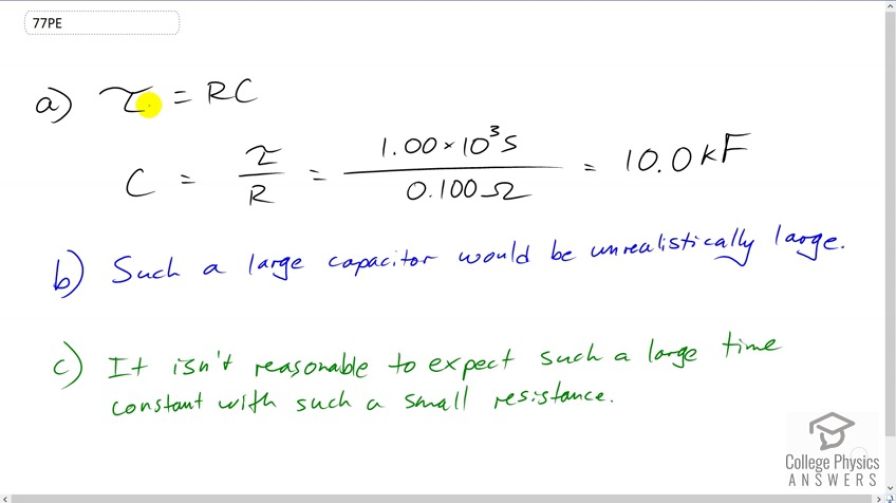
(a) Calculate the capacitance needed to get an time constant of with a resistor. (b) What
is unreasonable about this result? (c) Which assumptions are responsible?
Final Answer
- Such a large capacitor would be unrealistically large.
- It isn't reasonable to expect such a large time constant with such a small resistor.
Solution video
OpenStax College Physics for AP® Courses, Chapter 21, Problem 77 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We're identifying the capacitance needed to get a time constant of one times ten to the three seconds with a 0.1 Ohm resistor. And the time constant is resistance times capacitance so divide both sides by R and we get C as tau over R. So that's one times ten to the three seconds divided by 0.1 Ohms which is 10 Kilo Farads or ten times to the three Farads. And such a large capacitor is unrealistically large and would not be reasonable to expect such a large time constant of ten to the three seconds given such a small resistance of only 0.1 Ohms.
