OpenStax College Physics for AP® Courses, Chapter 21, Problem 30 (Problems & Exercises)

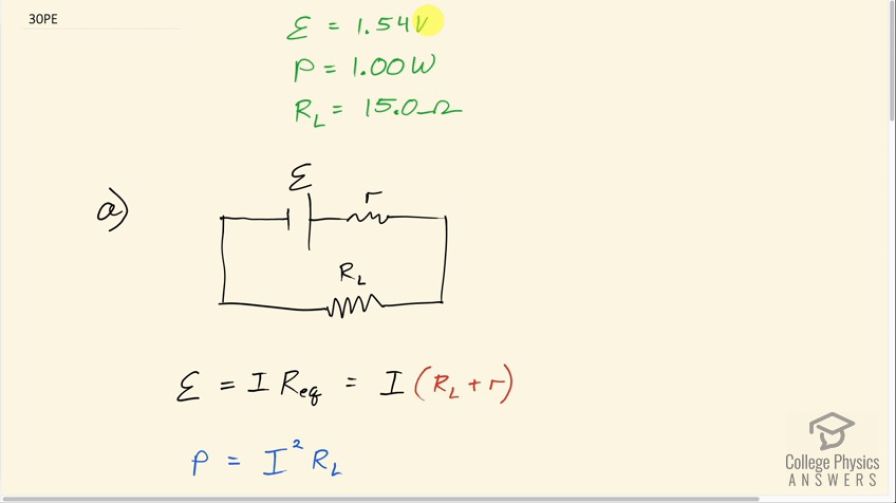
This is College Physics Answers with Shaun Dychko. We are going to find the internal resistance of this dry cell assuming that the emf is 1.54 volts, the power dissipated in the light bulb is 1.00 watt and the bulb has a resistance of 15.0 ohms. So the emf is the total voltage in this circuit; it is going to equal the current through the circuit multiplied by the total resistance and the total resistance is going to be the resistance of the light bulb plus the resistance of the internal resistance because they are connected in series, we can just add them together. Now we don't know what the current is but we can figure it out based on the power dissipated in the bulb and that power is going to be the current through the bulb squared multiplied by the bulb's resistance and we'll divide both sides by R L here to solve for I squared and then take the square root of both sides to solve for I. So I is the square root of the power dissipated in the bulb divided by the bulb's resistance. So the current is the square root of the power dissipated by the bulb divided by the bulb's resistance. So this is something we can substitute in for current in this formula. So the emf then is the square root of the power divided by bulb resistance multiplied by bulb resistance plus internal resistance and then we have to do some algebra to solve for little r. So we distribute this factor into the brackets and we have emf is R L times square root P over R L plus r times square root P over R L and this works out to square root P times R L because this is R L to the power of one divided by R L to the power of a half because that's what square root means with an exponent and so one minus a half is positive a half and so that's why we have R L in the square root sign here. And then we will subtract this square root PR L from both sides then we have R times square root P over R L equals emf minus square root PR L and then multiply both sides by the reciprocal of this so that's square root of R L over P multiply this by the square root of R L over P and it cancels on the left and ends up on the right side here; this is the same as dividing by square root P over R L but I think it just looks a little bit nicer because we don't have fraction in our denominator. So then we can substitute in numbers: we have internal resistance then is the square root of 15.0 ohms divided by 1.00 watt times 1.54 volts minus square root 1.00 watt times 15.0 ohms and that's negative 9.04 ohms. So that's what the algebra tells us but in part (b), we can say that this is a nonsensical answer... there's no such thing as a negative resistance so there's a problem with the numbers that we have been given in this question. So in part (c), we can see that if we have a 1.00 watt power dissipated through a resistance of 15.0 ohms, we can figure out what voltage must be across this load and that's the voltage from here to here, which is the terminal voltage of the battery in other words and so what voltage across that load? Well P is that voltage squared divided by R and then we multiply both sides by R and so R L for light bulb here. So V squared equals P times R L and then square root both sides to solve for V— this is the terminal voltage of the battery by the way— so that's the square root of power dissipated in the bulb multiplied by the bulb's resistance square root 1.00 watt times 15.0 ohms is 3.87 volts but that is impossible because this is greater than the emf of the battery so the internal resistance always reduces the terminal voltage when the battery is providing the current and so there's a problem with either this power being too big or this resistance being too big such that the terminal voltage needed is greater than the emf of the battery, which is not possible.