Question
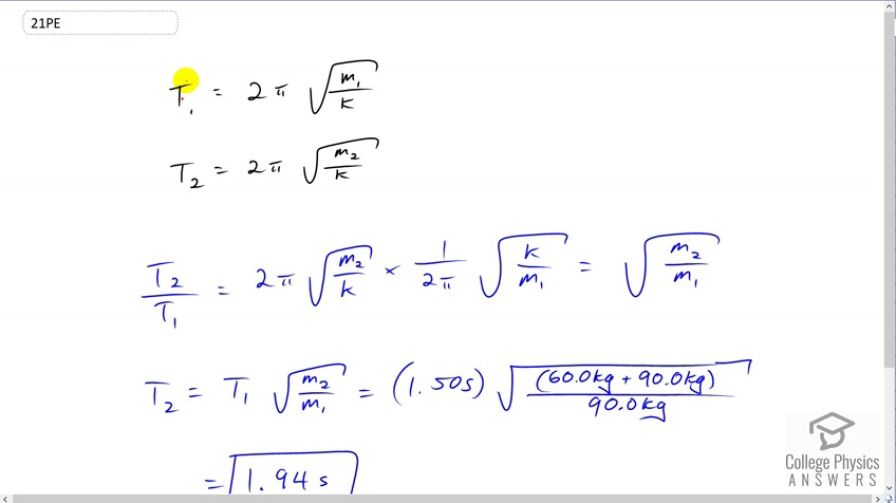
A 90.0-kg skydiver hanging from a parachute bounces up and down with a period of 1.50 s. What is the new period of oscillation when a second skydiver, whose mass is 60.0 kg, hangs from the legs of the first, as seen in Figure 16.47.
Final Answer
Solution video
OpenStax College Physics, Chapter 16, Problem 21 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The strings connecting the parachuters to the parachute are kind of elastic and causing the parachuters to bounce up and down. Now, in the first case there is one parachuter of mass 90 kilograms and we're given the period of oscillation of one and a half seconds. Then in the second case, the second parachuter of 60 kilograms is attached to the first parachuter of 90 and that makes a total mass, m two. We have to figure out what will the period be in that case. Well, period two divided by period one is going to be two pi times square root mass two over the spring constant of the strings in the parachute. Then we'll divide by T one which is the same as multiplying by its reciprocal. So we take the reciprocal of both factors. So we have one over two pi and then times by square root of k over m one. The square root k's cancel and so do the two pi's and we're left with square root m two over m one. So we multiply both sides by T one to solve for T two. We get T two is T one times square root m two over m one. So it's one and a half seconds period of oscillation when you have just the single parachuter by themselves, times the square root of the total mass of the two parachuters, 60 plus 90, divided by the mass of the single parachuter of 90 kilograms initially. This works out to 1.94 seconds will be the period of oscillation of the pair of parachuters together.
