Question
(a) What is the intensity in of a laser beam used to burn away cancerous tissue that, when 90.0% absorbed, puts 500 J of energy into a circular spot 2.00 mm in diameter in 4.00 s? (b) Discuss how this intensity compares to the average intensity of sunlight (about ) and the implications that would have if the laser beam entered your eye. Note how your answer depends on the time duration of the exposure.
Final Answer
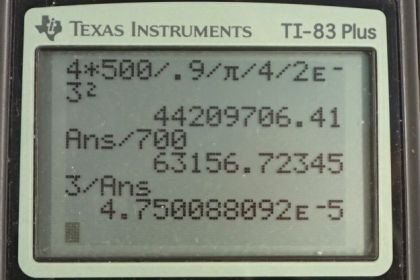
- The laser is more intense than the sun by a factor of 63200. Looking directly at the sun can damage the eye within seconds. The laser would deposit the same damaging amount of energy in the eye in a time that is shorter by a factor of , or approximately 0.50ms.
Solution video
OpenStax College Physics, Chapter 16, Problem 71 (Problems & Exercises)

vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. In this question we consider a laser beam that burns away cancerous tissue by putting 500 Joules of energy into a circular spot of two millimeters in diameter in four seconds with a 90 percent efficiency. So we're going to calculate what the intensity is. The power input divided by the area is the intensity and the power output which is the power delivered to the cancerous tissue we're told is 0.9 percent, or 0.9 times the power input, or 90 percent efficient in other words. We'll substitute P out over 0.9 in place of P in in our intensity formula because we'll solve for P in by dividing this by 0.9 and the other side by 0.9. We get P in is P out over 0.9. We substitute that in for P in and so intensity is the power delivered to the cancerous tissue divided by 0.9 times its area. The power delivered to the cancerous tissue is the energy delivered per time because we're given these two figures. This works out to W out over 0.9 t times area. Then substituting further, we have area -- it's a circular spot so its area is going to be pi times its diameter squared over four. This works out to four W out over 0.9 pi t diameter squared. So the intensity is four times 500 Joules of energy divided by 0.9 times pi times four seconds, times two millimeters written as two times ten to the minus three meters squared, giving an intensity of 4.42 times ten to the seven Watts per square meter. Comparing this to the intensity of the sun, this intensity of the laser divided by 700 watts per square meter gives a ratio of 63,200. Looking at the sun directly can damage the eye within a few seconds and this laser will deposit the same damaging amount of energy in a time that is shorter by a factor of one over 63,200. Or in other words if you took this and multiply it by three seconds I think, that works out to 0.05 milliseconds. So basically, instantly, this laser would damage the eye.