Solution video
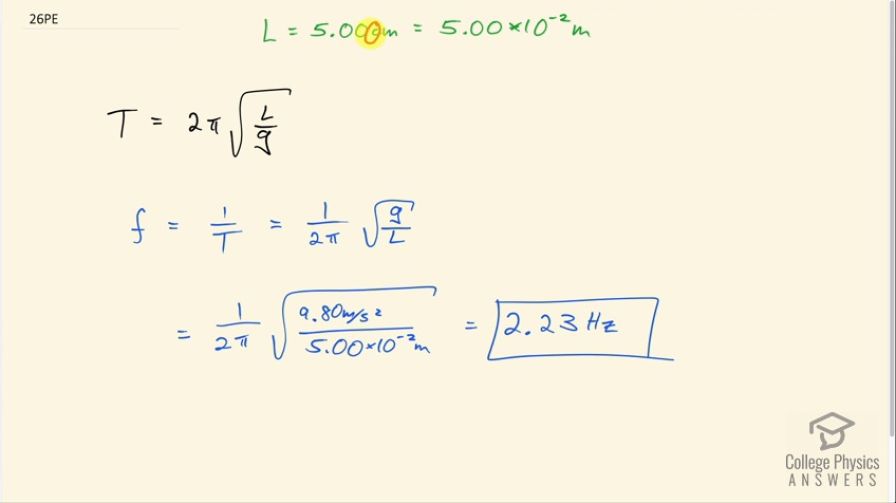
OpenStax College Physics, Chapter 16, Problem 26 (Problems & Exercises)
Calculator Screenshots
Comments
Could you please explain why on the calculator you did 1/2/pi, when the equation is written as 1/2*pi? I did the problem correctly but my answer was off by a factor of 10 and I'm confused as to where/why the conversion happens. Thank you!!
Hi bmudge, thank you for the question. I can't really guess why your answer is off by a factor of 10, but I can explain 1/2/pi.
1/2/pi is the same as 1/(2*pi). The calculator evaluates division left to right, so it evaluates 1/2 first, then divides that answer by "pi", which is equivalent to 1/(2*pi), but 1/2/pi has less calculator button pushing since there's no need to type in the brackets. It's just a personal preference. Keep in mind that 1/2*pi, however, is not the same, since that would mean "divide one by 2, and multiply that answer by pi", since there are no brackets to package the factors 2 and pi in the denominator.
Hope this helps,
Shaun


