Question
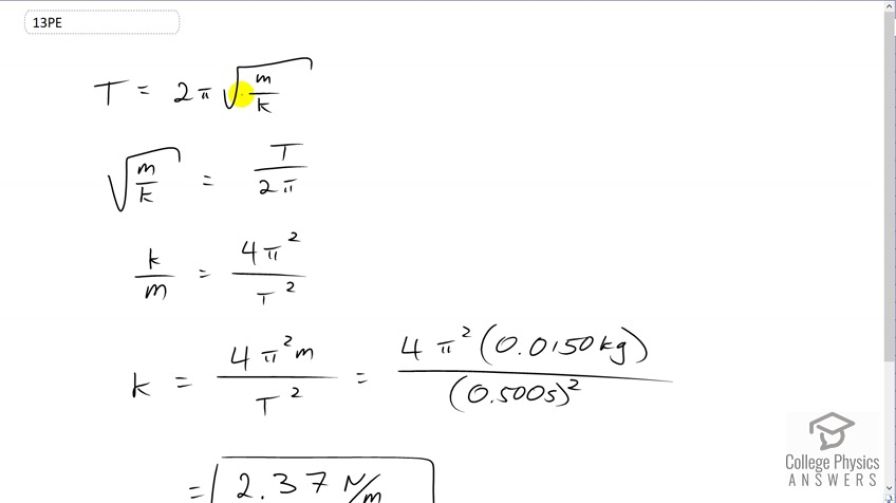
A type of cuckoo clock keeps time by having a mass bouncing on a spring, usually something cute like a cherub in a chair. What force constant is needed to produce a period of 0.500 s for a 0.0150-kg mass?
Final Answer
Solution video
OpenStax College Physics, Chapter 16, Problem 13 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. The period of an object moving with simple harmonic motion is two Pi times square root of the mass of the object divided by the spring constant. And so we have to solve for k. And so we're going to divide both sides by two Pi and then we have square root m over k equals period over two Pi. And then we'll raise both sides the exponent negative two. And the negative serves to flip the fractions and then there's going to be squared, as well. So, let's get rid of the square root sign at the left. And we're end up with k over m. Then the right hand side we have four Pi squared over period squared. Then, we'll multiply both sides by m and then we get the spring constant is four Pi squared m over period squared. So if four Pi squared times 0.015 kilograms divided by 0.5 second squared, giving us 2.37 newtons per meter is the spring constant.
