OpenStax College Physics, Chapter 16, Problem 39 (Problems & Exercises)

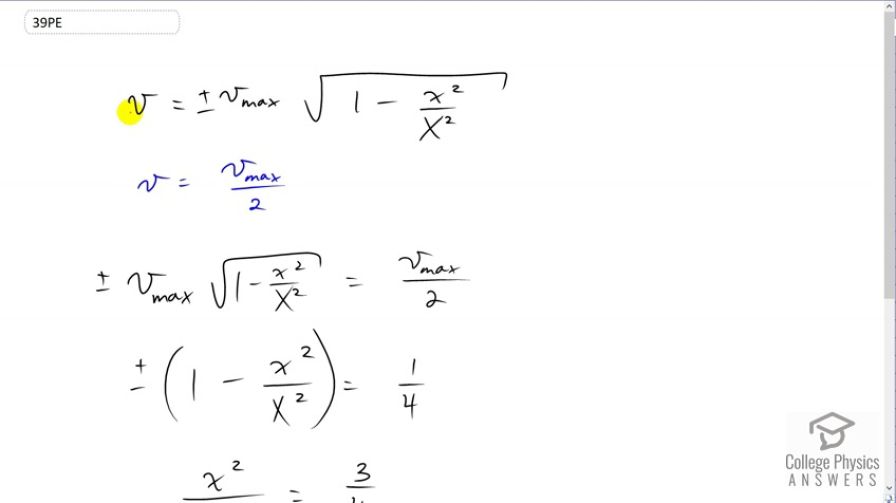
This is College Physic Answers with Shaun Dychko. In this question we're going to figure out at what positions the velocity of a simple harmonic oscillator will be half of the maximum velocity. So velocity for a simple harmonic oscillator is plus or minus the maximum times square root one minus the position squared divided by the amplitude squared. We're told the speed also is going to be half maximum. So equate these two which we do on this line, and we have plus or minus v max times square root one minus position squared divided by amplitude squared equals maximum speed divided by two. We can divide both sides by v max and we're left with this line. We're also squaring both sides. So this is one half squared makes one quarter and this squared makes one minus x squared over capital X squared. Then bring this to the right making it positive, take this to the left, giving one minus a quarter which is three quarters, then switch the sides around. We have position squared over amplitude squared equals three quarters. Then take the square root of both sides and we have to take the plus or the minus of the square root. That means the position divided by amplitude is plus or minus square root three over two. So this is the fraction of the amplitude will be the position where the velocity will be half maximum.