Question
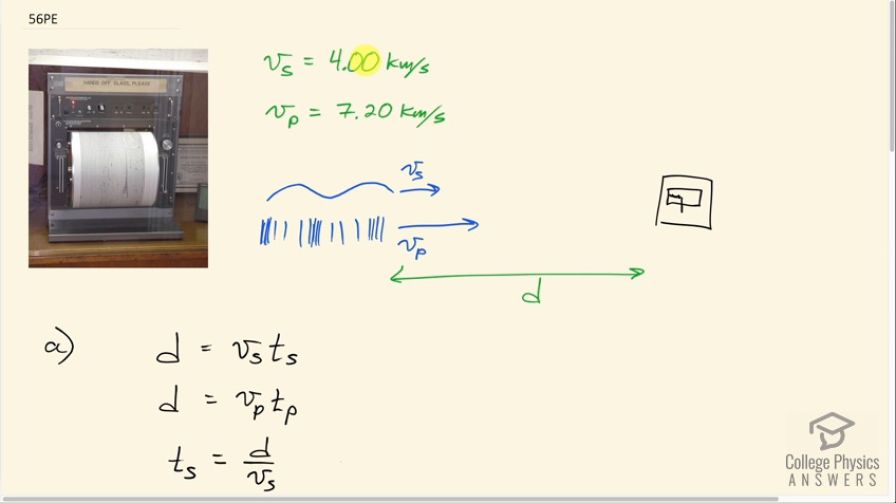
(a) Seismographs measure the arrival times of earthquakes with a precision of 0.100 s. To get the distance to the epicenter of the quake, they compare the arrival times of S- and P-waves, which travel at different speeds. Figure 16.48) If S- and P-waves travel at 4.00 and 7.20 km/s, respectively, in the region considered, how precisely can the distance to the source of the earthquake be determined? (b) Seismic waves from underground detonations of nuclear bombs can be used to locate the test site and detect violations of test bans. Discuss whether your answer to (a) implies a serious limit to such detection. (Note also that the uncertainty is greater if there is an uncertainty in the propagation speeds of the S- and P-waves.)
Final Answer
- This uncertainty is small enough that it's possible to be certain which country conducted a nuclear test. Uncertainty in wave propagation speed will create additional uncertainty.
Solution video
OpenStax College Physics, Chapter 16, Problem 56 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Two types of waves produced by an earthquake are transverse secondary waves which travel at 4.00 kilometers per second and longitudinal P waves which travel at 7.20 kilometers per second. The P waves get their name because they are primary or first; since they travel faster, they are the first ones that will be detected by a seismograph here whereas secondary waves travel slower and they are detected second. Now being able to measure the difference in the amount of time it takes for the waves to travel from the epicenter of the earthquake to the detector... that time difference will be used to measure the distance to the epicenter— which I guess is all the way here. Okay! So we can say that this distance is the speed of the secondary wave multiplied by the time it takes the secondary wave to reach the detector which we can't measure because we don't know when the earthquake begins so we can't figure out how much time it takes but we'll see that it doesn't matter in a second. This distance can also be written as the speed of the primary wave multiplied by the time it takes and we can rearrange each of these for their respective times so t S is d over v S and t P is d over v P. Now the thing that we can measure is the difference in the amount of time that each one takes so the difference between the secondary wave and the primary wave and that will be d over v S minus d over v P. and we can factor out the d and say that it's distance equals 1 over or distance time is 1 over v S minus 1 over v P. And in order to solve for d here which we wanna do since we wanna find out what is the uncertainty in distance based on knowing the uncertainty in this time difference measurement, we have to find a formula first for the distance. So we are gonna divide both sides by this bracket but that's gonna get messy so let's turn it into a single fraction and so we'll multiply top and bottom here by v P and multiply top and bottom here by v S and this works out to v P minus v S all over v Sv P so we have made a common denominator here between these two terms and written it as a single fraction and we have t S minus t P over here by the way. Now we multiply both sides by v Sv P over v P minus v S and that works out to d equals v Sv P times this time difference divided by v P minus v S. So the difference in the distance or the uncertainty in the distance measurement will be the uncertainty in the time difference measurement multiplied by all this same stuff and divided by this. So I mean I could have written a bit more detail here... you could say that the upper limit to the possible distance is v Sv P times t S minus t P upper divided by v P minus v S and then you know actual whatever distance that is this and then the difference in these distances d S minus d or d upper minus d is this whole thing minus this whole thing. Now you can factor out the v Sv P over v P minus v S from both of these terms and you end up with this expression here where we have t S minus t P upper limit minus t S minus t P. Okay! So we can plug in numbers at this point so we have the secondary wave speed multiplied by the primary wave speed multiplied by this uncertainty in our time difference measurement of 0.100 seconds divided by the difference in wave speeds and that is 0.900 kilometers. Now this uncertainty is small enough that it's possible to be certain which country conducted a nuclear test although changes in the wave propagation speed or uncertainty in that wave propagation speed will certainly add much more to our uncertainty in this distance.
