OpenStax College Physics, Chapter 16, Problem 31 (Problems & Exercises)

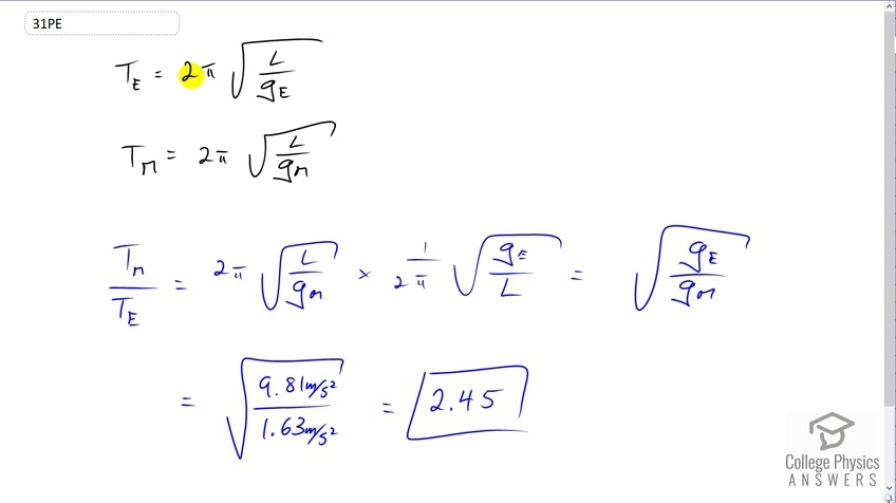
This is College Physics Answers with Shaun Dychko. We're going to find the ratio of the period of a pendulum on the moon versus on earth. So on the earth the period of the pendulum will be two pi times square root of its length divided by the acceleration due to gravity on the earth. Then on the moon, the period of the pendulum will be two pi times square root of the same length because it's the same pendulum, but now divided by acceleration due to gravity of the moon which is why there is a subscript m for moon there on the g. So T m divided by T e is going to be two pi times square root L over g m and then multiply by the reciprocal of the period on earth. So we flip each of the factors here. So we have one over two pi times square root g e over L. So the square root L's cancel and so do the two pi's and we're left with square root g e over g m. So the period of the moon divided by the period on earth is going to be the square root of the ratio of the accelerations due to gravity. So that's square root of 9.81 meters per second squared, acceleration due to gravity on the earth, divided by 1.63 meters per second squared, acceleration due to gravity on the moon. We get a ratio of periods is going to be 2.45. So the period of this pendulum on the moon will be 2.45 -- let me put it this way. The period on the moon will be greater than the period on the earth by a factor of 2.45.