Question
A pendulum with a period of 2.00000 s in one location () is moved to a new locatio where the period is now 1.99796 s. What is the acceleration due to gravity at its new location?
Final Answer
Solution video
OpenStax College Physics, Chapter 16, Problem 29 (Problems & Exercises)
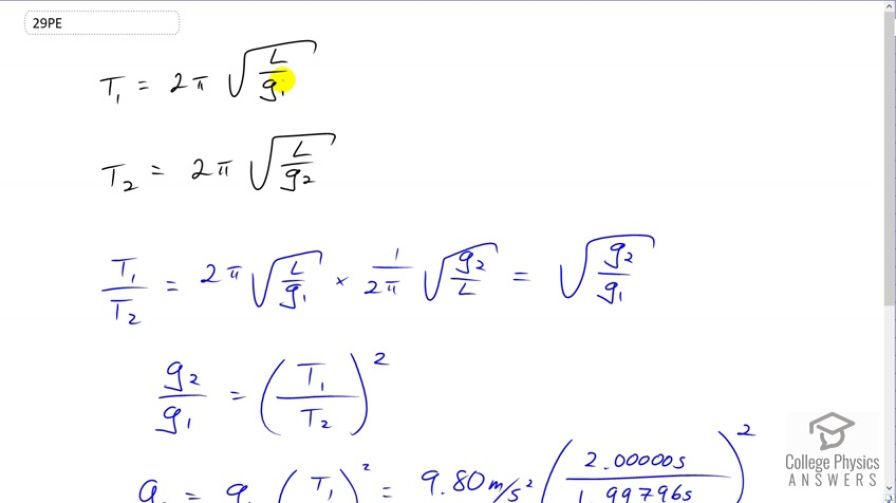
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. In the first location, the period of this pendulum will be T one and that'll be two pi times square root of the length of the pendulum divided by the acceleration due to gravity in that first location and we'll call it g subscript one. In the second location, period two will be two pi times square root of the same length so there is no need for a subscript on the L, but it's going to be divided by g two in this case which is a different acceleration due to gravity in this second location. Now, T one divided by T two, is going to be two pi times square root L over g one, and then dividing by T two is the same as multiplying by the reciprocal of it which means flip every factor here. So we're going to multiply by one over two pi times square root g two over L. The two pi's cancel and so do the square root L's and we're left with square root g two over g one. Now, if we square this side and then square this as well, we get g two over g one is T one over T two squared. Then multiply both sides by g one to solve for g two the acceleration due to gravity in location two. So it's going to be g one times T one over T two squared. So that's 9.80 meters per second square, acceleration due to gravity in the first location, times two seconds, period in the first location, divided by 1.99796 seconds, period in the second location, and square that fraction. You get 9.82 meters per second squared is the acceleration due to gravity in the second location. There are three significant figures here because this number has three significant figures.
