Question
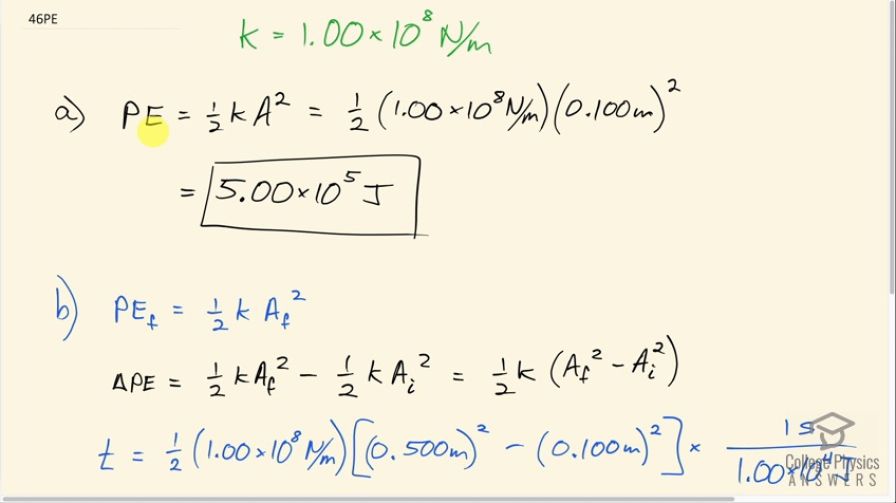
Engineering Application: A suspension bridge oscillates with an effective force constant of . (a) How much energy is needed to make it oscillate with an amplitude of 0.100 m? (b) If soldiers march across the bridge with a cadence equal to the bridge’s natural frequency and impart
of energy each second, how long does it take for the bridge’s oscillations to go from 0.100 m to 0.500 m amplitude?
Final Answer
Solution video
OpenStax College Physics, Chapter 16, Problem 46 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. Soldiers are walking across a suspension bridge that has a force constant of 1.00 times 10 to the 8 newtons per meter and the question is how much energy is needed to make it oscillate with an amplitude of 0.100 meters? So when the bridge is stretched to its full amplitude that means all of its mechanical energy is stored as potential energy in the spring so to speak in the stretching of the ropes or whatever cables are suspending it. So all of its energy is going to be tied up in potential energy and the formula for that is one-half times the spring constant times the amount of stretching which in this case is the amplitude since it's the maximum amount of stretch squared. So that's one-half times 1.00 times 10 to the 8 newtons per meter times 0.100 meters of stretching squared which is 5.00 times 10 to the 5 joules. Part (b) says suppose that when soliders are marching across the bridge, they are giving energy to the bridge at a rate of 1.00 times 10 to the 4 joules every second and so how long does it take to get the bridge's oscillations from 0.100 meter amplitude to 0.500 meters amplitude? So the final potential energy will be one-half times the spring constant times the final amplitude squared and so we can find the change in energy starting from the initial and then find the difference between that and the final potential energy and we can factor out the one-half k here from both terms. So we have one-half k times final amplitude squared minus initial amplitude squared and then the time it will take to give that much energy will be whatever that ΔP is which is everything from here to here—that's ΔP— multiplied by 1 second for every 1.00 times 10 to the 4 joules this is the rate at which energy is given to the bridge but I have taken the reciprocal of it in order to have the joules from this part highlighted in yellow canceling with these jewels in the denominator and they will leave us with an answer in seconds. This works out to 1200 seconds which I convert into minutes and so it's 20.0 minutes.
