OpenStax College Physics, Chapter 16, Problem 70 (Problems & Exercises)

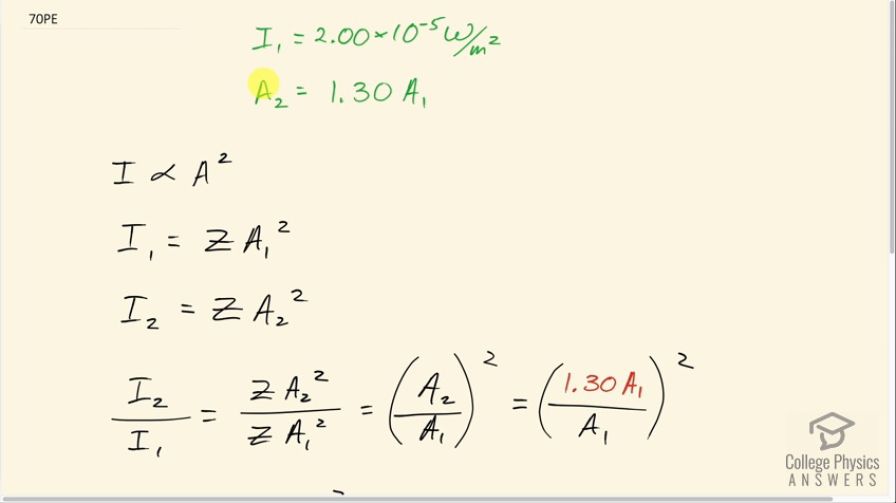
This is College Physics Answers with Shaun Dychko. The intensity of a sound wave in the first case is 2.00 times 10 to the minus 5 watts per square meter and we are told that the amplitude of the sound is increased such that it will now be 30 percent more than the amplitude was when the original intensity was measured. So to say that the amplitude is gonna be 30 percent more is to say that A 2 will be 1.30 times A 1. So we know that intensity is proportional to amplitude squared which means that I 1—the first intensity— equals some constant of proportionality— whatever it is doesn't matter— multiplied by A 1 squared. because when you say something is proportional to something else, you are saying that it equals some factor multiplied by that thing. So that means I 1 equals some factor Z times A 1 squared; I 2 will be the same factor multiplied by A 2 squared and it's the same factor because it's the same microphone being used with the same area and so on. Now I 2 divided by I 1 will be ZA 2 squared divided by ZA 1 squared and the Z's cancel meaning we have A 2 divided by A 1 squared and A 2 we know is 1.30 times A 1 so we make that substitution in place of A 2 and the A 1's cancel leaving us with 1.30 squared is the ratio of I 2 over I 1. So that means I 2 equals 1.30 squared times I 1. So that's 1.30 squared times 2 times 10 to the minus 5 watts per square meter which is an intensity of 3.38 times 10 to the minus 5 watts per square meter.