Question
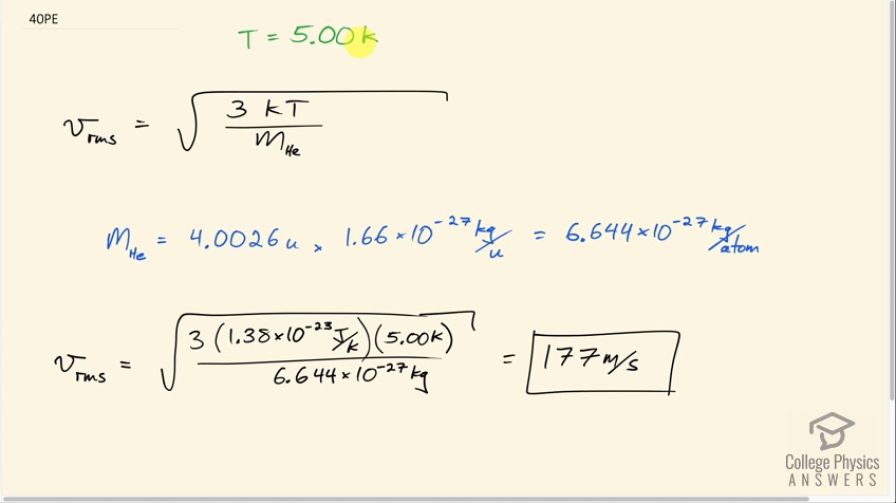
Average atomic and molecular speeds () are large, even at low temperatures. What is for helium atoms at 5.00 K, just one degree above helium’s liquefaction temperature?
Final Answer
Solution video
OpenStax College Physics, Chapter 13, Problem 40 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Prev
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This question is asking us for the root mean squared velocity of a helium atom at a temperature of five Kelvin. So V RMS we're told is the square root of three times Boltzmann's constant times the absolute temperature divided by the mass. And the mass of a helium atom if you put in to Google, it will give you 4.0026 atomic mass units and then you have to look up the conversion between atomic mass units to kilograms which is 1.66 times 10 to the minus 27 kilograms per atomic mass unit. We end up with 6.644 times 10 to the negative 27 kilograms per atom of helium. So the V RMS the is the square root of three times 1.38 times 10 to the minus 23 joules per Kelvin, that's Boltzmann's constant, times the temperature of five Kelvin divided by this mass of a single atom and that is 177 meters per second.
Comments
I don't know why, but every time I plug in those calculations into my calculator I get 1.76E -25?
nevermind!