OpenStax College Physics, Chapter 13, Problem 20 (Problems & Exercises)

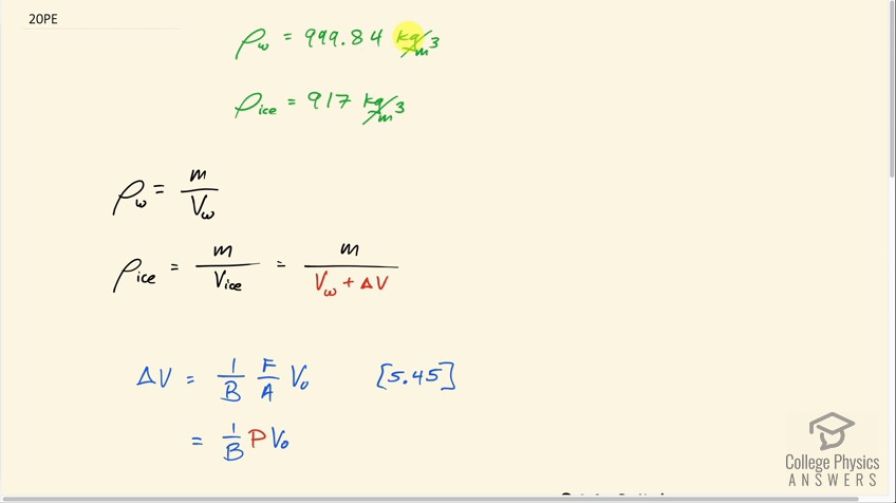
This is College Physics Answers with Shaun Dychko. The density of liquid water at zero degrees Celsius is 999.84 kilograms per cubic meter and the density of the same substance but in solid form - ice, in other words - is 917 kilograms per cubic meter at zero degrees Celsius. And this question is asking: how much pressure would be needed to squish this solid ice so much such so you have the same density as liquid water at zero degrees Celsius? And we're ignoring the fact that pressure actually changes the melting point of substances. We'll ignore that fact here. So, the density of water is the mass of water divided by the water's volume. And the density of ice is the same mass because it is the same quantity, the same amount of the matter but divided by a new volume - the volume of ice. And this volume is the original volume of the water plus some extra volume due to the ice freezing and expanding as a result. Now this change in volume due to freezing is going to be the change that we need to cause in the opposite direction using pressure. So we need to squish the ice this amount which will be the same as that amount using pressure. So, from chapter five, equation 45 says that the change in volume of a substance is one over its bulk modulus multiplied by force per area, which is pressure, multiplied by its original volume. And so I'm substituting P in place of F over A here. And so we are going to come up with an expression for Delta V based on these densities and then we'll say Delta V from that analysis of densities is this Delta V here and then this contains a pressure factor and then we can solve for it. So, the ratio of densities, water divided by density of ice is the density of water mass divided by volume of water divided by the density of ice, but I'm writing it as a multiplication, though, because I find it confusing to divide a fraction by a fraction. So I'm multiplying by the reciprocal of the denominator. So the density of ice is M over V W plus delta V, and so I'm going to instead multiply it by the flipped version of that. So I multiply by V W plus delta V over M. The M's cancel and then we have V W plus delta V over V W. So that is the ratio of the densities. But we have numbers for these densities. And so, we can also say that it's 999.84 kilograms per cubic meter, density of water divided by 917 kilograms per cubic meter, density of ice, and then solve for delta V. And multiply both sides by V W and then after doing that multiplying then subtract V W from both sides and we have this line here. So delta V then is this ratio of densities times the original volume of water minus the volume of water. Now, this delta V is the same magnitude as this delta V which is pressure times original volume divided by both modulus. And instead of a V 0, I'm going to write V W here. This is the original volume of the water. And we'll solve this for P by multiplying both sides by both modulus divided by volume of water. Now, V W is the common factor between these two terms. So we can factor it out and then cancel with this denominator V W. That becomes one there. And we have B multiplied by the ratio of densities minus one is going to be the pressure. So we look up in table 5.3 the bulk modulus of water which is 2.2 times 10 to the nine newtons per square meter multiplied by the density of water divided by the density of ice minus one and that is 2.0 times 10 to the eight newtons per meter squared is the pressure that will be needed. And this is about 2,000 atmospheres of pressure. So it's a huge pressure.