OpenStax College Physics, Chapter 13, Problem 60 (Problems & Exercises)

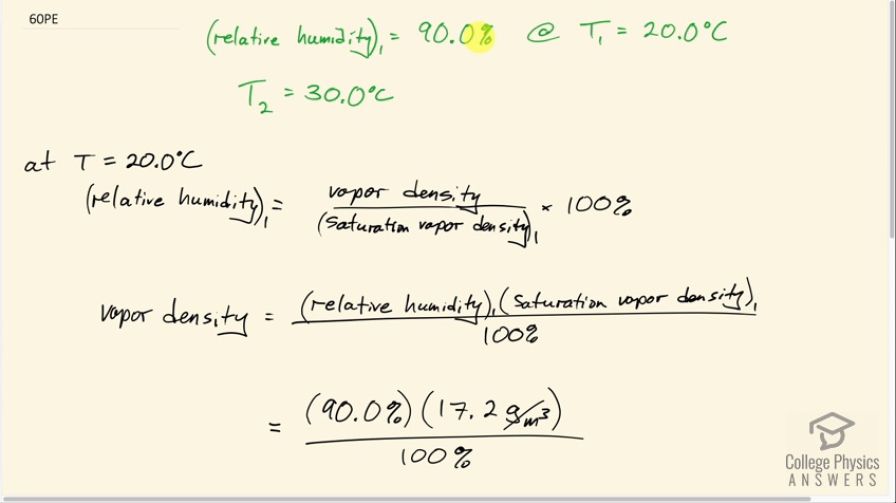
This is College Physics Answers with Shaun Dychko. We have a relative humidity which we call number one at 20 degrees Celsius of 90% and at some other temperature T two 30 degrees Celsius. We are asked to find out what is the relative humidity then assuming the same vapor density. So there's no water taken away or removed from the air between these two different temperatures. So at 20 degrees Celsius we have relative humidity number one is the vapor density that we're going to have to find divided by the saturation vapor density at this temperature of 20 degrees times 100% And we can solve for vapor density by multiplying both sides by the saturation vapor density and then also divide both sides by 100% and then you get this line here. So vapor density is the relative humidity number one times saturation vapor density number one. And to find the saturation vapor density at 20 degrees Celsius we look at table 13.5 and follow the temperature column down to 20 and the saturation vapor density in grams per cubic meter is 17.2. So we substitute that in here times 90% divided by 100% and that gives us 15.48 grams per cubic meter. So that's the vapor density. Now take that same vapor density and consider a temperature of 30 degrees Celsius, what would the relative humidity be then? So we take that vapor density divided by the new saturation vapor density at 30 degrees Celsius, and following the temperature column to 30, we see that that's 30.4. There's higher saturation vapor density at this higher temperature and times that by 100% and we get 50.9% is the relative humidity. So there's a dramatic change in the relative humidity given the same amount of water in the air for two different temperatures that are different by only ten degrees - 20 to 30.