OpenStax College Physics, Chapter 13, Problem 66 (Problems & Exercises)

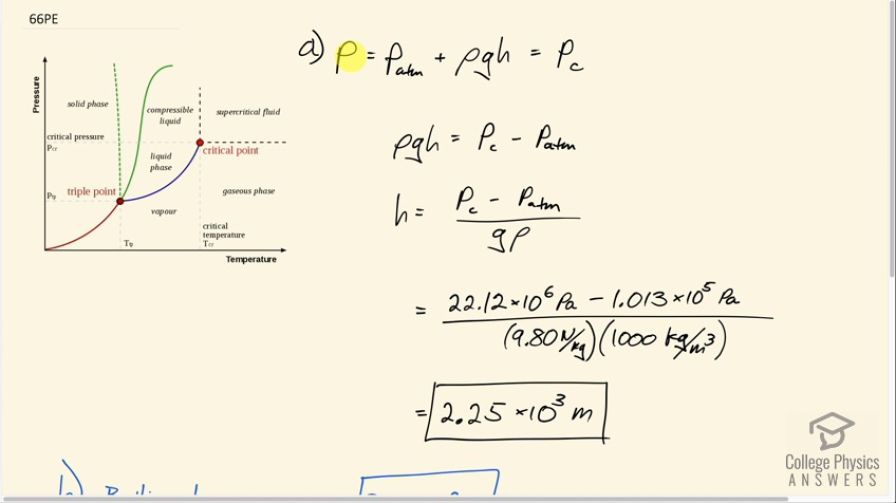
This is College Physics Answers with Shaun Dychko. We're going to find the depth in fresh water at which the absolute pressure will be greater than the critical pressure for water. So the critical pressure we can look up in table 13.3 and it's 22.12 times 10 to the 6 pascals. And so that critical pressure will happen with this body of water having a surface at sea level it'll have some atmospheric pressure there, and then add to that the pressure due to the vertical column of water which is given by the formula density times 'g' times the height of the column. So we're going to solve for h by subtracting atmospheric pressure from both sides and then dividing both sides by g and rho. And so the depth then is the critical pressure minus atmospheric pressure divided by gravitational field strength times density of fresh water. And so this is 22.12 times 10 to the six pascals minus 1.013 times 10 to the 5 pascals divided by 9.8 times a thousand kilograms per cubic meter. And that's 2.23 or 2.25 times 10 to the 3meters. And this isn't really that unreasonably deep. I know we're dealing with fresh water here but the Mariana trench is 10 kilometers deep and this is two kilometers deep. So critical pressures do exist in the everyday world. I mean, if you want to call the deep ocean the everyday world and being above the critical pressure means that it'll be in a compressible liquid state. Now, part B asks us what will the boiling temperature be at this depth, and the answer is the critical temperature which we can also look up here which is 374.3 degrees Celsius. And so part C asks: is a significantly higher temperature needed to boil water at a greater depth? And the reason they're asking that is because as pressure goes up as you go up higher on this vertical axis of this pressure/ temperature diagram and for water we follow this dotted green line here separating the solid phase and the liquid phase, whereas for most substances you have this solid green curve. But we can ignore this solid green curve because that's for things that are not water-like. Water is unusual because its density increases in a solid state whereas most substances have a decreased density in the solid state. So as you get to higher pressures, normally the boiling point which is the temperature at which you change from liquid to vapor or gas phase increases. So at this pressure the boiling point is at this temperature whereas at this pressure x, the temperature at which it boils will be this temperature. And so the question is asking: so now at a greater and greater pressure will you need a greater and greater temperature still to boil? And the answer is no. Strange things happen after this critical point, and so above this critical temperature it doesn't matter what the pressure is. It'll be gas no matter what. So at this really small pressure here we have a gas phase at this temperature which is to the right of this critical temperature here. And then at this really high pressure deeper down in the ocean, likewise, you still have a gaseous phase. It's called a supercritical fluid but the thing that makes it strange is that it's not really that much different from the gas phase. And so you get this gas-like supercritical fluid which is not a liquid certainly at any pressure when you have a temperature greater than the critical temperature. So here is a compressible liquid and here's a supercritical fluid and you go from one to the other at all pressures so long as you're to the right of this critical temperature. Okay. There we go!