Question
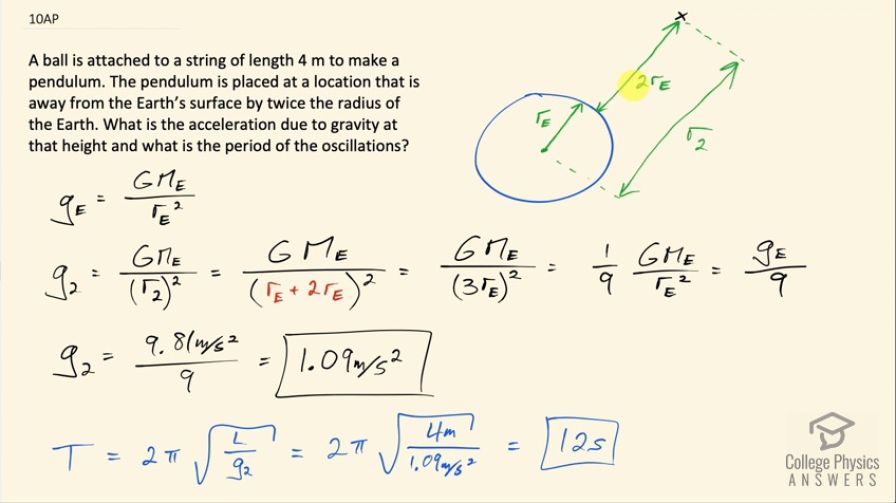
A ball is attached to a string of length 4 m to make a pendulum. The pendulum is placed at a location that is away from the Earth’s surface by twice the radius of the Earth. What is the acceleration due to gravity at that height and what is the period of the oscillations?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 16, Problem 10 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A pendulum is suspended from a string of length 4 meters and it's located at a position two times the Earth's radius from the surface of the Earth so this means the distance from the center of the Earth is that two times Earth's radius from the surface plus the distance to the center of the Earth from the surface. So the total distance r 2 then is going to be radius of the Earth plus 2 times radius of the Earth for a total of 3 times the radius of the Earth. Okay! So acceleration due to gravity on the Earth's surface is this gravitational constant times the mass of the Earth divided by the Earth's radius squared and the gravitational acceleration at position two here is going to be GM e—the same as before— but now divided by this new distance r 2 squared and r 2 is 3 times Earth's radius and this works out to 1 over 9 times GM E over r E squared and this is acceleration due to gravity on the surface. So it's g—that we are used to— 9.81 meters per second squared divided by 9 and so that works out to 1.09 meters per second squared. The period of a pendulum position there is gonna be 2π times the square root of the length of the pendulum divided by the acceleration due to gravity there. So that's 2π times square root of 4 meters over 1.09 meters per seconds squared and that is 12 seconds.
