OpenStax College Physics for AP® Courses, Chapter 16, Problem 36 (Problems & Exercises)

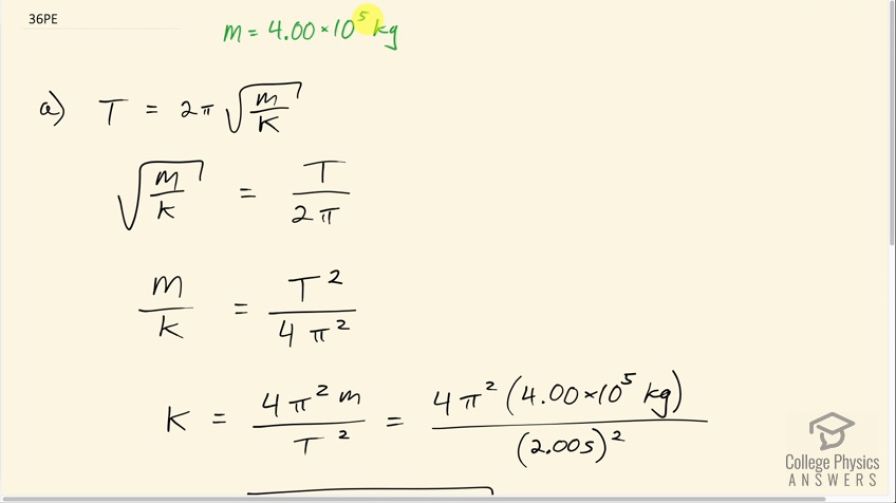
This is College Physics Answers with Shaun Dychko. The mass on the top of the City Group building in New York City is 4.00 times 10 to the 5 kilograms and it's connected by springs that will make this mass move back and forth in the opposite direction to how the wind is pushing the building so that, you know, the net effect on the building is nil since the mass is what's moving instead of the building. So we have the period is 2π times square root of the mass divided by spring constant and we wanna find out what k is and we are given the period of 2.00 seconds. So we have square root m over k is T divided by 2π and then we take the square of both sides and then we get m over k equals T squared divided by 4π squared and then we solve for k by multiplying both sides by k and also by 4π squared over T squared and then switch the sides around as well so we have k equals 4π squared m over T squared. So that's 4π squared times 4.00 times 10 to the 5 kilograms divided by 2.00 seconds squared and that's 3.95 times 10 to the 6 newtons per meter. And then we are asked how much potential energy is stored in a spring with this kind of spring constant if it's displaced 2.00 meters from equilibrium? So the formula for that is one-half times spring constant times displacement from equilibrium squared. So that's one-half times 3.9478 times 10 to the 6 newtons per meter times 2.00 meters squared which is 7.90 times 10 to the 6 joules.