Question
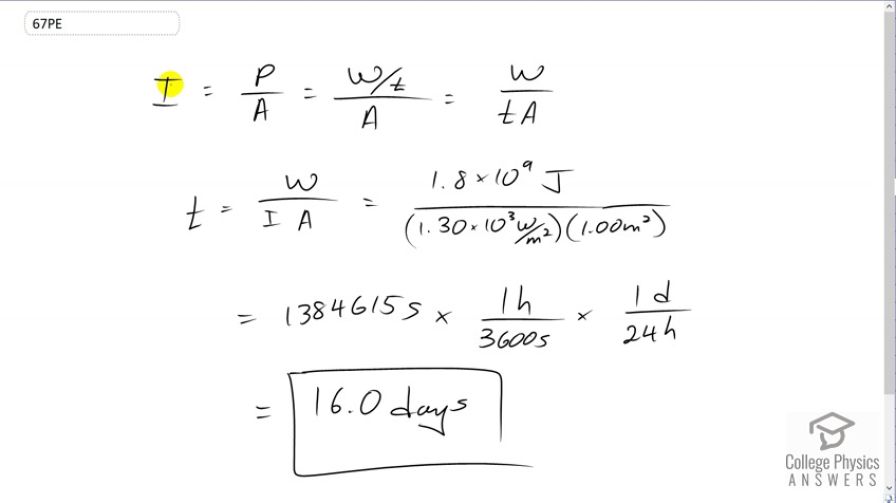
Energy from the Sun arrives at the top of the Earth’s atmosphere with an intensity of How long does it take for to arrive on an area of ?
Final Answer
Note: this answer should have two significant figures on account of the 2 sig. figs. in the energy given in the question (. The video mistakenly shows three significant figures in the final answer.
Solution video
OpenStax College Physics for AP® Courses, Chapter 16, Problem 67 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
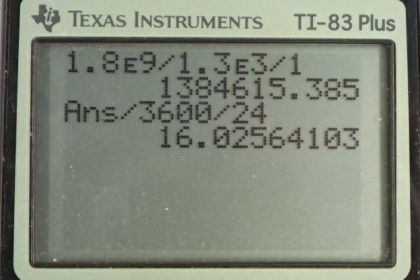
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We're going to calculate the amount of time it takes for a certain amount of energy from the sun to impact an area of one square meter. So intensity is power divided by area and power is the rate at which energy is being delivered, so work divided by time in other words. This can be written as work divided by time, times area. Now, we'll solve for t by multiplying both sides by t over intensity and we get that t is work over intensity times area. So that's 1.8 times ten to the nine Joules of energy, divided by the intensity of the sun's radiation which is 1.3 times ten to the three Watts per square meter, times one square meter, giving this amount of time in seconds which we'll convert into days in order to have a better intuition about what this time means. So multiply by one hour for every 3600 seconds then multiply by one day for every 24 hours and we get 16.0 days.