Question
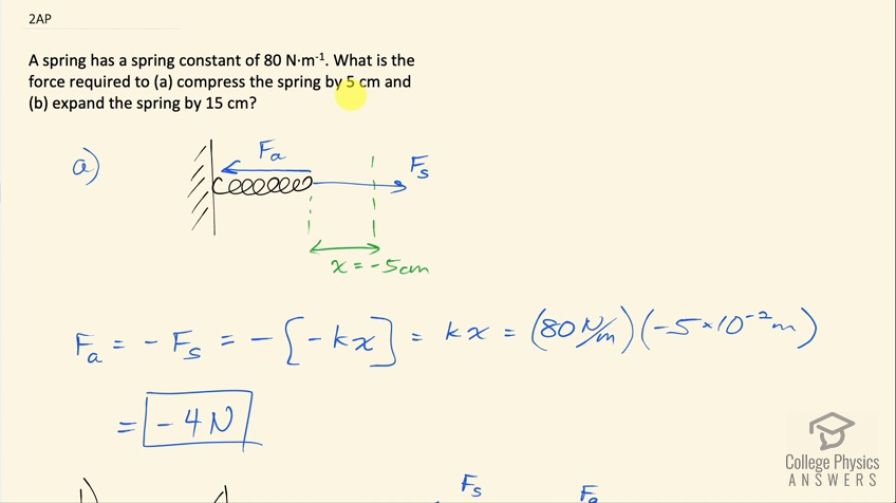
A spring has a spring constant of force required to (a) compress the spring by 5 cm and (b) expand the spring by 15 cm?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 16, Problem 2 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A spring has a spring constant of 80 newtons per meter and what is the force required to compress the spring 5 centimeters or to expand it by 15 centimeters? So this is the force applied on the spring in part (a) and it's going to be compressing it so its equilibrium position is here and it's now going to be 5 centimeters compressed compared to its equilibrium position— we'll call that negative 5 centimeters negative to indicate a compression— and it's asking what is the force required to do this compression so it's asking for the applied force. Hooke's law gives us the spring force which is going to be trying to return the spring back to its equilibrium position so the applied force is in the opposite direction to the spring force and that means it's the negative of the spring force so the applied force then is the negative of negative kx—this being the force due to the spring— the negative sign indicating that the spring force... restoring force direction is in the opposite direction to the change in length x. So this makes positive kx so that's 80 newtons per meter times the negative 5 times 10 to the minus 2 meters—compression— and that is negative 4 newtons. In part (b), the applied force will be to the right here and the spring force will be to the left the spring force again we are trying to return the spring back to its equilibrium position here and it's being displaced 15 centimeters to the right here— this is an expansion— and so the change in length is 15 centimeters positive. So the applied force then is kx, which is 80 newtons per meter times positive 15 times 10 to the minus 2 meters and that is 12 newtons.
