Question
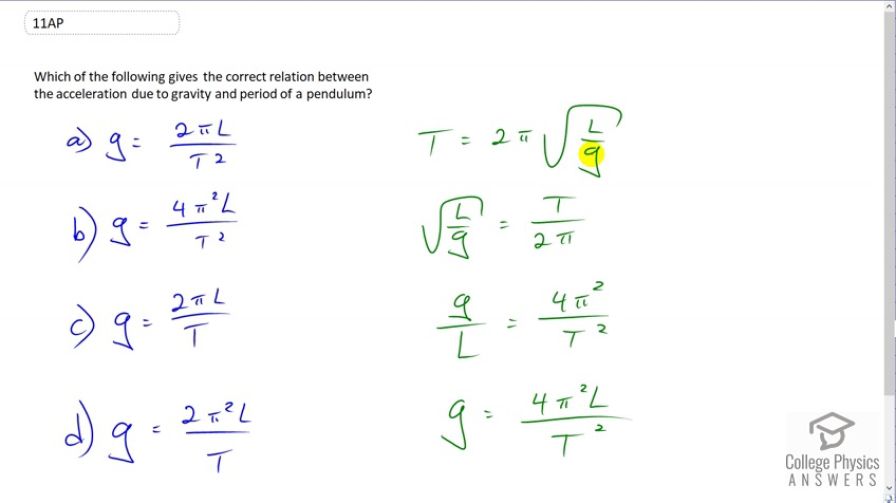
Which of the following gives the correct relation between the acceleration due to gravity and period of a pendulum?
  1. g=2πLT2g=\dfrac{2\pi L}{T^2}
  2. g=4π2LT2g=\dfrac{4\pi^2 L}{T^2}
  3. g=2πLTg=\dfrac{2\pi L}{T}
  4. g=2π2LTg=\dfrac{2\pi^2 L}{T}
Question by OpenStax is licensed under CC BY 4.0
Final Answer

(b)

Solution video

OpenStax College Physics for AP® Courses, Chapter 16, Problem 11 (Test Prep for AP® Courses)

OpenStax College Physics, Chapter 16, Problem 11 (AP) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Next Chevron right
Video Transcript
This is College Physics Answers with Shaun Dychko. The period of a pendulum is two Pi times square root of its length divided by the acceleration to the gravity. We're going to solve for g by dividing both sides by two Pi giving us square root L over g is T two Pi. And then we'll raise both sides to the exponent negative two. The negative sign serves to flip both fractions and then afterwards we square them. So this makes g over L on the left and it makes four Pi squared over T squared on the right. And then we'll solve for g by multiplying both sides by the length. So g is four Pi squared L over T squared. And this is option b.