Question
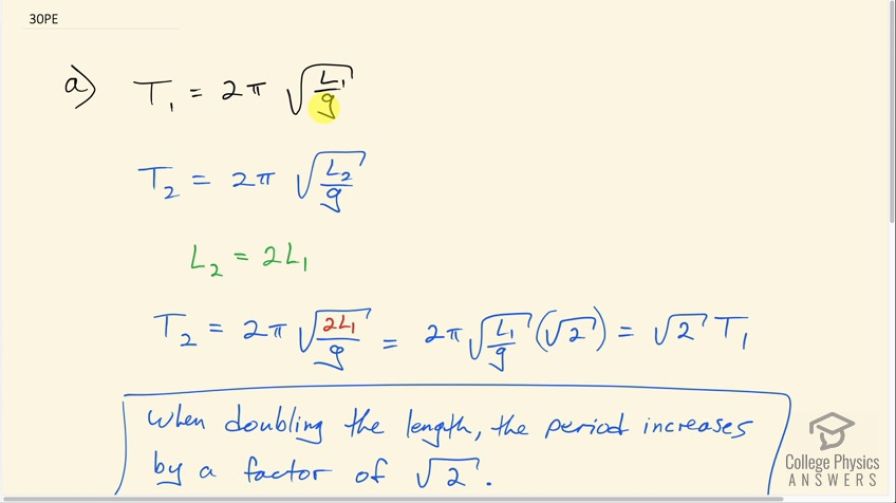
(a) What is the effect on the period of a pendulum if you double its length?
(b) What is the effect on the period of a pendulum if you decrease its length by 5.00%?
Final Answer
- When doubling the length, the period increases by a factor of
- The period decreases by 2.5% when decreasing the length by 5%.
Solution video
OpenStax College Physics for AP® Courses, Chapter 16, Problem 30 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A pendulum has an initial period of 2π times square root of its original length L 1 over g and then it's gonna have some new period T 2 which is 2π times square root of its new length L 2 over g and we are told that L 2 is 2 times L 1. So we'll substitute in 2L 1 in place of L 2 here and this works out to 2π times square root L 1 over g times square root of 2. Now all of this is T 1 and so we can replace this with T 1 here and I guess we should write that in red to show that it's a replacement and we see that T 2 then is square root 2 times T 1. So when doubling the length, the period increases by a factor of square root 2 which is about 1.4. In part (b) we are supposed to suppose that length two is 5 percent less than length one so a reduction of 5 percent means multiply L 1 by a factor of 0.9500. So we plug in 0.9500L 1 in place of L 2 and this works out to 0.9500 times T 1 and if you take the square root of 0.950, it's 0.975 so the period decreases by 2.5 percent because this is 2.5 less than 100. So 2.5 percent when decreasing the length by 5 percent.
