OpenStax College Physics for AP® Courses, Chapter 16, Problem 28 (Problems & Exercises)

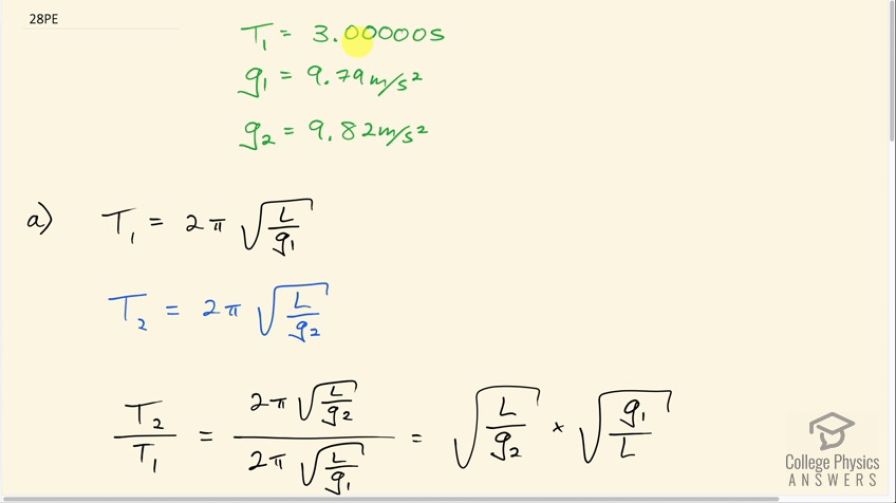
This is College Physics Answers with Shaun Dychko. A pendulum has a period of 3.00000 seconds and we are given a precision of five decimal places here and it has this period and a reach where the acceleration due to gravity is 9.79 meters per second squared. Each of these pieces of information are labeled with a subscript 1 to say this is a period in location 1 on Earth. And then we take the same pendulum to a different location on Earth where the acceleration due to gravity is g 2 which is 9.82 meters per second squared and the question is what will the period be there? So the period at location one is 2π times the square root of L over g 1 and the L does not get a subscript one because it's going to be the same pendulum in the two locations and so there's no need to distinguish the lengths... they are going to be the same length since it is the same pendulum. So period two is going to be 2π times square root L over g 2. So there are a variety of different ways we can do the algebra to solve for T 2; we could solve this equation for T 1 for L and then make a substitution but then that would be a bit messy because you would have a lot of stuff getting plugged into the top of a fraction that's within a square root sign so instead we are going to divide these two equations. So T 2 divided by T 1 is going to be 2π times square root L over g 2 all over 2π times square root L over g 1. The 2π's cancel and dividing this fraction by this fraction can be done in a more clear way I think by multiplying by the reciprocal of the denominator. So instead of dividing by square root L over g 1g 1 over L. And the square root L's cancel and we are left with T 2 over T 1 is square root g 1 over g 2 and then we can solve for T 2 by multiplying both sides by T 1 and we get T 2 is T 1 times square root g 1 over g 2. So that's 3.00000 seconds times square root of 9.79 meters per second squared divided by 9.82 meters per second squared which is 2.99541 seconds. Now strictly speaking perhaps we should have only three significant figures in our answer but what I think the question is intending to show is the change in period and we wouldn't be able to show that if we have only three significant figures because it would be 3.00. So there we go! Part (b) it says, you know, why do we need so many digits of precision here so many significant figures, in other words? Well, we can show how the period depends on changes in g and T 2 is T 1 times square root g 1 over g 2 as we said over here and we'll suppose that g 2 is some factor x multiplied by g 1 and so we make a substitution xg 1 in place of g 2 and then the g 1's cancel and we are left with T 2 is T 1 over square root x which is to say that changing g by a factor of x, as we did here, results in a change in period of 1 over square root x. And so the 1 over square root x is gonna be a smaller change than well... 1 over than just the factor change that happened to g itself. So maybe an example with numbers is better so if x is 1.01 which represents an increase of 1 percent then period would decrease by a factor of 1 over square root of 1.01 which is 0.9950 which is a reduction of 0.50 percent. So whereas g increased by 1 percent, period is decreasing by less than that factor, only 0.50 percent. So period is not very sensitive to changes in g so that's why lots of digits are needed to see the effect of changing g.