Question
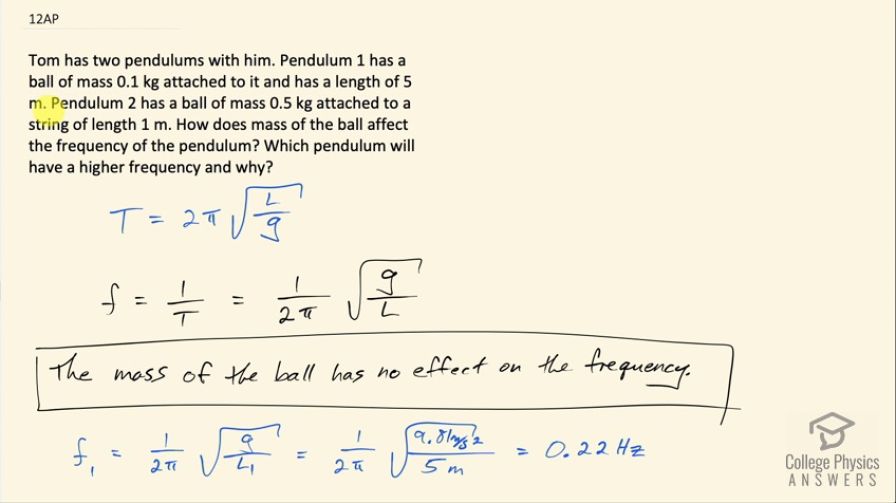
Tom has two pendulums with him. Pendulum 1 has a ball of mass 0.1 kg attached to it and has a length of 5 m. Pendulum 2 has a ball of mass 0.5 kg attached to a string of length 1 m. How does mass of the ball affect the frequency of the pendulum? Which pendulum will have a higher frequency and why?
Final Answer
The mass of the ball has no effect on the pendulum frequency. Pendulum 2 has a higher frequency due to a shorter length.
Solution video
OpenStax College Physics for AP® Courses, Chapter 16, Problem 12 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. We consider two pendulums: number one has a mass of 0.1 kilograms attached to it and a length of 5 meters and pendulum two has a 0.5 kilogram mass and a length of 1 meter and so how does the mass of a ball affect the frequency of the pendulum is the first question? Well, period of a pendulum is 2π times the square root of its length divided by acceleration due to gravity and the frequency is the reciprocal of a period so that's 1 over 2π times square root of g over L and we can see that m does not appear in this formula anywhere so the mass of the ball has no effect on the frequency in the same way that the mass of something free falling through frictionless circumstances will have the same acceleration regardless of its mass and in the same way, a pendulum has no mass-dependence in its frequency because frequency, you know, sort of represents the speed of falling. Okay! So frequency of the first pendulum is 1 over 2π times square roof of g over the first length so that's 1 over 2π times 9.81 meters per seconds squared divided by 5 meters and that's 0.22 hertz. Frequency two is 1 over 2π times square root of 9.81 meters per seconds squared divided by 1 meter and that is 0.50 hertz. So we see that frequency two is greater than frequency of pendulum one and that's because pendulum 2 has a shorter length.
