Question
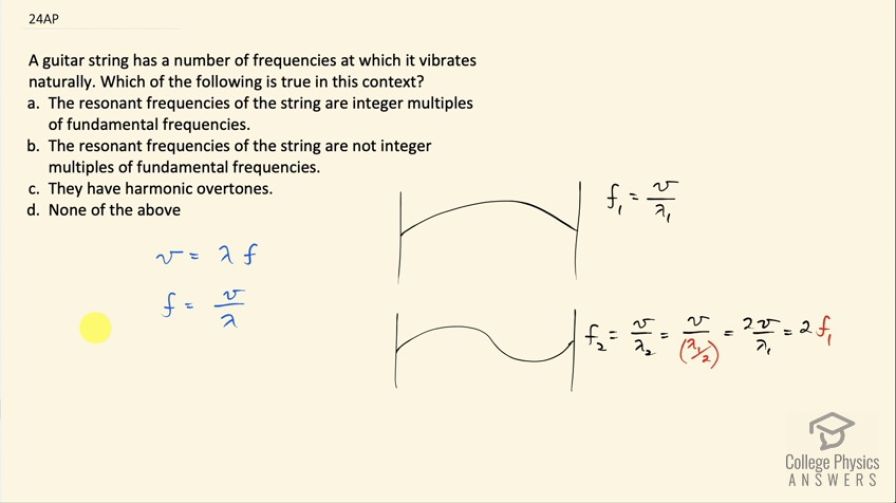
A guitar string has a number of frequencies at which it vibrates naturally. Which of the following is true in this context?
- The resonant frequencies of the string are integer multiples of fundamental frequencies.
- The resonant frequencies of the string are not integer multiples of fundamental frequencies.
- They have harmonic overtones.
- None of the above
Final Answer
(a)
Solution video
OpenStax College Physics for AP® Courses, Chapter 16, Problem 24 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Video Transcript
This is College Physics Answers with Shaun Dychko. A guitar string has a number of frequencies at which it vibrates naturally and which of the following is true? So we are gonna talk about the overtones and the fundamental frequency and we know that the speed of a wave is its wavelength multiplied by its frequency and we can divide both sides by λ to solve for f and the reason we are doing this is because we can visualize the wavelengths pretty well for a guitar string and then we'll use this to translate what we can see into a frequency. So this would be the fundamental frequency for a guitar string— we have a single antinode— and that will be frequency one and then it will be some wave speed, which is determined by the mass of the string and its tension divided by this fundamental wavelength λ 1. The next possible wavelength would be this one because we need to have nodes at both ends because the string is attached there and then this is the next waveform that fits with a node at each end and we'll call this λ 2—this wavelength— and this wavelength is half what the old wavelength was and we can substitute λ 2 then with λ 1 over 2 and this works out to 2v over λ 1 and v over λ 1 is f 1 we see that f 2 is 2 times f 1 and so on and so on we can add a third picture here and have three antinodes and then we can say that f 3 is going to be v over λ 1 over 3 and that's going to be 3 times f 1 and we can see that the frequencies are going to be integer multiples of the fundamental and so the answer is (a).