Question
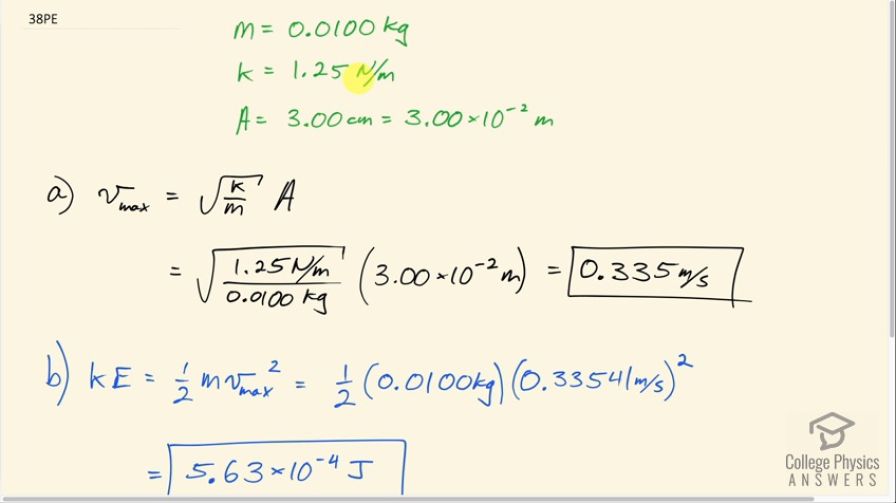
A novelty clock has a 0.0100-kg mass object bouncing on a spring that has a force constant of 1.25 N/m. What is the maximum velocity of the object if the object bounces 3.00 cm above and below its equilibrium position? (b) How many joules of kinetic energy does the object have at its maximum velocity?
Final Answer
Solution video
OpenStax College Physics for AP® Courses, Chapter 16, Problem 38 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
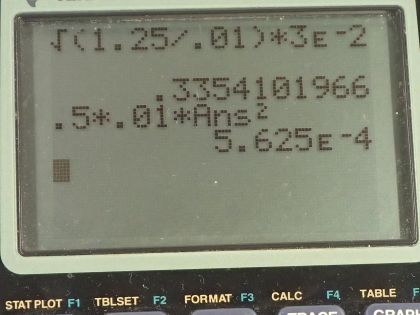
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A novelty clock has a mass of 0.0100 kilograms bouncing on a spring with spring constant 1.25 newtons per meter and the maximum displacement from equilibrium, or its amplitude in other words, is 3.00 centimeters which is 3.00 times 10 to the minus 2 meters. We are asked to find out what is the maximum speed of this mass and that is the square root of the spring constant divided by the mass times its amplitude. So that's square root of 1.25 newtons per meter divided by 0.0100 kilograms times 3.00 times 10 to the minus 2 meters which is 0.335 meters per second. The kinetic energy it has during its maximum speed is one-half times its mass times its maximum speed squared. So that's one-half times 0.0100 kilograms times the answer for part (a) written with lots of digits squared and that's 5.63 times 10 to the minus 4 joules.