Question
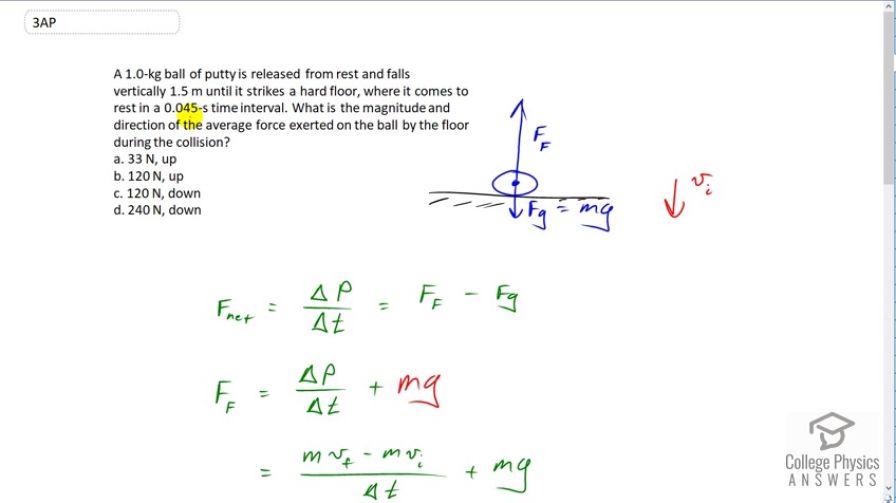
A 1.0-kg ball of putty is released from rest and falls vertically 1.5 m until it strikes a hard floor, where it comes to rest in a 0.045-s time interval. What is the magnitude and direction of the average force exerted on the ball by the floor during the collision?
- 33 N, up
- 120 N, up
- 120 N, down
- 240 N, down
Final Answer
(b)
Solution video
OpenStax College Physics for AP® Courses, Chapter 8, Problem 3 (Test Prep for AP® Courses)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. This ball of putty impacts the hard floor after it falls 1.5 meters. It takes 0.045 seconds for it to come to rest. The question is what is the force exerted by the floor on the putty. So when it hits the floor, there is going to be two forces on it, one being gravity downwards mg, and the other one being the force due to the floor. So we'll write down Newton's Second Law which is that the net force is the change in momentum of the putty divided by the time. That's going to be the friction force minus the force due to gravity. Now we'll add Fg to both sides and we'll solve for the force due to the floor. Did I say friction force? That's not friction force, that's force due to the floor. Okay. So force due to the floor is change in momentum over time plus gravity. The change in momentum is the mass times the final velocity of the putty minus mass times its initial velocity, divided by time. This initial velocity is the velocity that it has when it just begins contacting the floor. It is dropped from rest one and a half meters up here but that's not the initial velocity we're speaking of here. We're speaking of the velocity when it just begins to touch the floor. So final velocity is zero because it comes to rest and so this equation simplifies to m times bracket negative v i over delta t plus g when we factor out the common factor m. Now, we don't know what this velocity is so we can figure it out though and once we plug in for that, we'll be able to find the force due to the floor. So the kinetic energy that the putty has when it just begins to contact the floor is equal to the gravitational potential energy it has initially. We can solve for v by dividing both sides by m, multiplying both sides by two and then taking the square root of both sides. When taking the square root you can take the plus or the minus square root. In this case we'll take the negative square root because we can see that the putty is going downwards and we'll take that to be the negative direction, down. So we have v is negative square root of two g h and so we substitute for that in our formula for the force due to the floor. So it's m times bracket negative formula times negative from v square root two gh all over delta t, plus g. So that's one kilogram times square root of two times 9.8 meters per second squared times 1.5 meters, divided by 0.045 seconds plus 9.8 which gives 130 newtons. Now, in our list of options here we don't see 130 newtons. I think that's because in coming up with these options the person who created this question forgot to include gravity or they assumed that the gravity was negligible. If you were to take away 9.8 from this and just multiply one by this term here I think you would get 120 probably and so our answer is going to be B because certainly this force due to the floor is upwards in order to arrest the downward velocity of the putty.
