Question
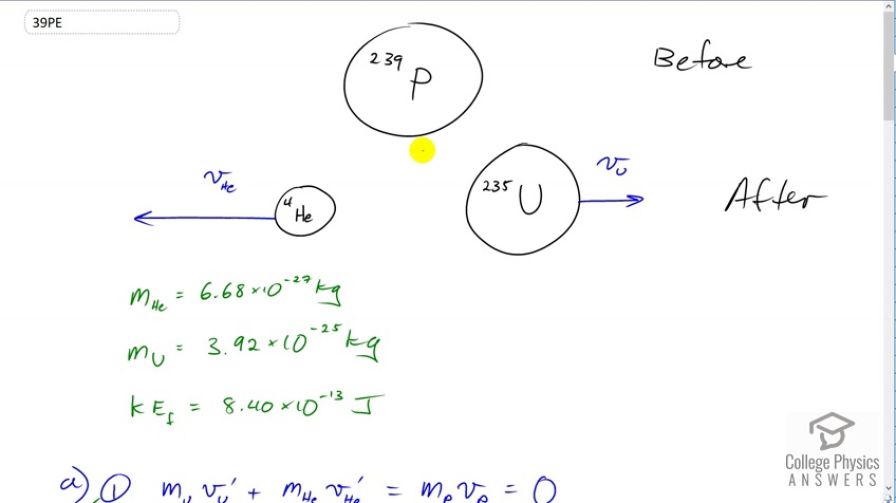
One of the waste products of a nuclear reactor is plutonium-239 . This nucleus is radioactive and decays by splitting into a helium-4 nucleus and a uranium-235 nucleus , the latter of which is also radioactive and will itself decay some time later. The energy emitted in the plutonium decay is and is entirely converted to kinetic energy of the helium and uranium nuclei. The mass of the helium nucleus is , while that of the uranium is (note that the ratio of the masses is 4 to 235). (a) Calculate the velocities of the two nuclei, assuming the plutonium nucleus is originally at rest. (b) How much kinetic energy does each nucleus carry away? Note that the data given here are accurate to three digits only.
Final Answer
a)
b) ,
Solution video
OpenStax College Physics for AP® Courses, Chapter 8, Problem 39 (Problems & Exercises)
vote with a rating of
votes with an average rating of
.
Calculator Screenshots
Video Transcript
This is College Physics Answers with Shaun Dychko. A plutonium atom is initially at rest and so it has zero momentum. After some time it will spontaneously decay into two different nuclei, a helium four nucleus and it'll be going one direction and the uranium 235 nucleus which will be going the opposite direction such that the momenta of these two combined will add up to the momentum the system had before which was zero. So given that the uranium is much more massive than the helium, its velocity will be of shorter arrow here, or shorter vector and it'll have smaller magnitude compared to the velocity of the helium nucleus since the product of velocity and mass for each of these things has to be of equal magnitude, but opposite directions. Okay. So the helium mass is given and the uranium mass is given. They have a total kinetic energy after the decay of 8.4 times ten to the minus thirteen joules. So we'll write down in an equation form what I said before, that the final momenta will be zero because it's going to equal the initial momentum which is zero since the velocity of the plutonium is zero. We also can write expressions for the kinetic energy, one half mass of the uranium times the velocity of the uranium after decay, squared, plus one half mass of the helium times the velocity of the helium after decay, squared, equals 8.4 times ten to the minus thirteen joules. So we have a system of two equations and we need to solve for the unknowns, that being the velocity of the uranium and the velocity of the helium after decay. So we'll rearrange equation one to solve for velocity of the uranium and we'll do that by moving this term to the right hand side by subtracting it from both sides, then divide both sides by the mass of the uranium. So we have mass of the uranium, it's going to be negative mass of the helium times velocity of the helium prime over mass of the uranium. That is something we can substitute into equation two into our kinetic energy formula. So we'll substitute for v u prime and replace it with this fraction. So we can re-write equation two as this, maybe we might call this equation two version b. So that's one half mass uranium times this expression for velocity of the uranium prime, and then so on and so on. Everything else is copied there and then we'll multiply both sides by two -- let's erase this because it might get confusing there. So multiply both sides by two and that makes 16.8 times ten to the minus thirteen joules on the right side and then on the left side the fractions one halves cancel on both terms. I squared this bracket and then multiplied by the mass u factor there and so on the denominator there's mass of the uranium to the power of one because it would be squared due to the square here. But then by multiplying by that it's mass u to the power of one. Then in the numerator we have mass of the helium squared times velocity of the helium after decay squared. Then add to that this term but it does not have one half factor any more so I think I'd cancel by multiplying by two and then, we want to solve for velocity of the helium prime. We'll factor this common factor out. This is velocity of the helium prime squared, and then we multiply this term by mass of uranium divided by mass of uranium so that we can write this whole thing over a common denominator m u which isn't necessary to do but I think it just looks nicer. Then we multiply both sides by the reciprocal of this factor. So m u over m he squared plus mass helium mass uranium, and do that to both sides, then it cancels on the left side and then on the right hand side it's there. Then we'll also take the square root of both sides. When taking the square root of both sides of an equation, you can choose whether to take the positive or the negative. Now because I've drawn the helium going towards the left and to the right is traditionally taken as positive, that means this velocity of helium you expect to be negative. So that's why I put a negative there. So we have velocity of the helium after decay is going to be negative, the square root of 16.8 times ten to the minus thirteen joules of kinetic energy, times the mass of the uranium, 3.92 times ten to the minus twenty-five kilograms, divided by the mass of the helium squared, 6.68 times ten to the minus twenty-seven kilograms, and then plus the product of the helium and uranium masses. Then we get negative 1.57 times ten to the seven meters per second will be the velocity of the helium. Now the velocity of the uranium we can get by rearranging this momentum formula by taking this term to the right side that makes it negative mass helium times velocity helium. Then we divide both sides by mass of the uranium. So, we have that here. We have negative 6.68 times ten to the minus twenty-seven kilograms multiplied by this velocity that we just found for the helium, negative 1.57252 times ten to the seven meters per second and I'm keeping lots of digits here in order to avoid intermediate rounding error. Then we divide by the mass of the helium and we get 2.68 times ten to the five meters per second for the velocity of the uranium. Sure enough it is much, much slower than the helium. So we're always doing a bit of reality check whenever we get answers. If this number had been bigger than the velocity of helium we would have known that there must have been some mistake there. Okay. So the kinetic energy of the helium is one half of its mass times its velocity squared, so I've plugged in numbers there to get 8.26 times ten to the minus thirteen joules and the kinetic energy of the uranium is one half mass of the uranium times the velocity of the uranium after decay, squared. This gives 1.41 times ten to the minus fourteen joules.


