Question
Two cars (A and B) of equal mass have an elastic collision. Prior to the collision, car A is moving at 15 m/s in the +x-direction, and car B is moving at 10 m/s in the –x-direction. Assuming that both cars continue moving along the x-axis after the collision, what will be the velocity of car A after the collision?
  1. same as the original 15 m/s speed, opposite direction
  2. equal to car B’s velocity prior to the collision
  3. equal to the average of the two velocities, in its original direction
  4. equal to the average of the two velocities, in the opposite direction
Question by OpenStax is licensed under CC BY 4.0
Final Answer

(b)

Solution video

OpenStax College Physics for AP® Courses, Chapter 8, Problem 25 (Test Prep for AP® Courses)

OpenStax College Physics, Chapter 8, Problem 25 (AP) video thumbnail

In order to watch this solution you need to have a subscription.

Start free trial Log in
vote with a rating of votes with an average rating of .
Chevron left Prev
Next Chevron right
Video Transcript
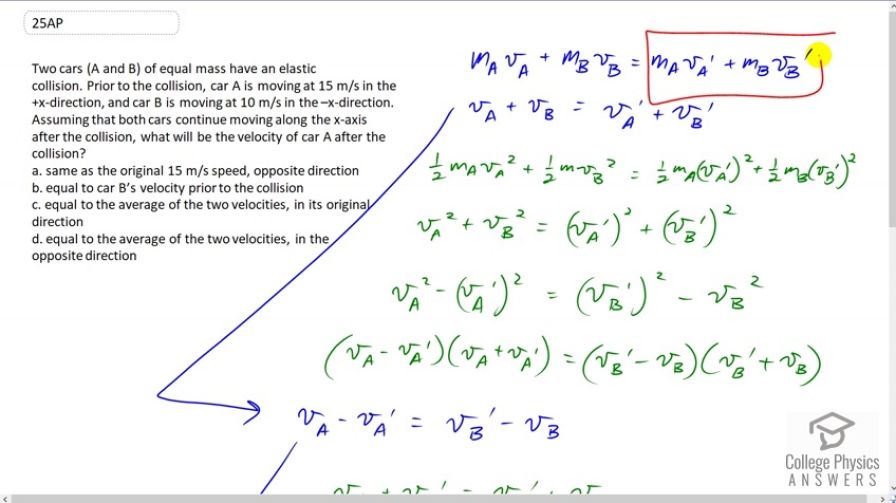
This is College Physics Answers with Shaun Dychko. Conservation of momentum says that mass A times vA plus mass B times vB equals the total momentum after the collision. Since the masses are the same we can cancel all these m's and we get vA plus vB equals vA prime plus vB prime. Now we're going to look at conservation of kinetic energy which is what we have in an elastic collision and again we can cancel all the m's and cancel the one halves and we get this expression. Then we can move this term to the left side and move this one to the right side and we get the difference of squares which we can then factor out. So we get vA minus vA prime times vA plus vA plus vA prime, equals vB prime minus vB, times vB prime plus vB. The reason that's useful is because we can rearrange this conservation of momentum formula and create one side of the equation saying vA minus vA prime and the other side saying vB prime minus vB and these two are equal. So since they are equal these are common factors as it turns out so they can be canceled. So this conservation of energy formula reduces to vA plus vA prime equals vB prime plus vB. We can rearrange that to solve for vA prime which is ultimately what this question is asking us for, what will the velocity of car A be after the collision. Well, we don't have an answer here because we don't know what the velocity of car B will be after the collision, so we consider the conservation of momentum again and solve for vB prime. It is vA minus vA prime plus vB. We substitute that in for vB prime here and that's what is shown in this line. We get vA prime equals vA minus vA prime, plus vB, plus vB minus vA. The vA's cancel and we move this to the left side by adding it to both sides so we get two vA prime equals two vB. Divide both sides by two and you get that the velocity of cart A after the collision will equal the velocity of cart B before the collision. So the answer is B.